In the equation $h_t(x,y|...) = ...$, can anyone explain me why the first derivatives of the marginal distributions are included? $H_t$ is a distribution function and $h_t$ its density function.
Using the relation between distribution and density function I can't get it to make sense.
$$f(x,y)=\frac{\partial ^2 F(x,y)}{\partial x \partial y}\,.$$
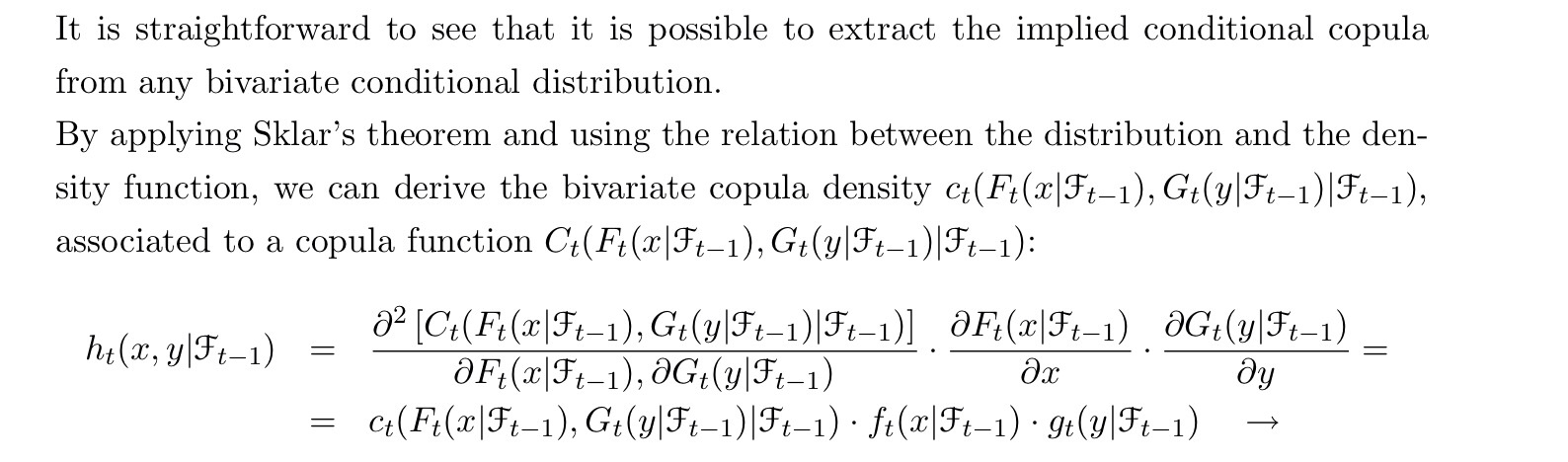
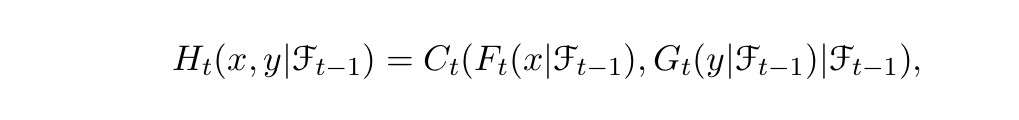By definition, $F$ is the distribution function of a bivariate random variable $(X,Y)$ when
$${\Pr}(a\lt X \le b,\, c\lt Y \le d) = F(b,d) - F(a,d) - F(b,c) + F(a,c)$$
for all real numbers $a\le b,\, c\le d$.
Suppose $F$ is continuously twice-differentiable. Let $f(x,y) = \frac{\partial ^2}{\partial x \partial y}F(x,y)$ be its mixed second derivative. Being a continuous function, it is integrable, and--applying Fubini's Theorem--we may exploit the Fundamental Theorem of Calculus to integrate twice:
$$\eqalign{ \iint_{(a,b]\times(c,d]} f(x,y) dxdy &= \int_a^b \left(\int_c^d \frac{\partial}{\partial y}\left(\frac{\partial}{\partial x}F(x,y)\right) dy \right)dx \\ &= \int_a^b \left(\frac{\partial}{\partial x}F(x,d) - \frac{\partial}{\partial x}F(x,c)\right)dx \\ &= F(b,d) - F(a,d) - \left(F(b,c) - F(a,c)\right) \\ &= {\Pr}(a\lt X \le b,\, c\lt Y \le d). }$$
This exhibits $f$ as a valid density function for $(X,Y)$.
A little extra care is needed when $f$ is not continuous (but only integrable), but even so, this case demonstrates why the two partial derivatives appear.
