I found this definition of central limit theorem in the book: Intro to probability and statistics using R:
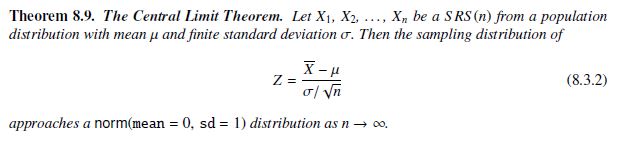
I thought the sample mean $\bar X$ itself followed the normal distribution and not the calculated quantity as shown.
I found this definition of central limit theorem in the book: Intro to probability and statistics using R:

I thought the sample mean $\bar X$ itself followed the normal distribution and not the calculated quantity as shown.
Well the sample mean $\bar{X}$ approaches a normal distribution with mean $\mu$ and variance $\frac{\sigma^2}{n}$, i.e. $\bar{X}\sim N(\mu,\frac{\sigma^2}{n}) $ however if you standardize the sample mean (by writing it in the form in the question), you find that approaches a Normal distribution with mean $0$ and variance $1$, i.e. $Z \sim N(0,1)$.
It's a very common mistake - which I make sometimes myself - to have the non-constant on the right hand side of the arrow: $$f_n\to \mathcal{N}\left(\mu,\frac{\sigma^2}{n}\right)$$, where $f_n$ is the probability distribution of $\bar X$.
Since your right hand side is changing, it's difficult to prove statements about this convergence relation, and I mean impossible by difficult.
Hence, we want to have the constant right hand side to apply our convergence proving tricks, e.g. $\mathcal{N}(0,1)$ would be a non-changing distribution. So, we formulate the convergence statement using it: $$Z=\frac{\bar X-\mu}{\sigma/\sqrt n}\sim\tilde f_n$$, where $\tilde f_n$ - sequence of distributions (functions), which converges to the standard normal: $$\tilde f_n\to\mathcal{N}(0,1)$$
This one we can handle: the right hand side is not changing, only the left hand side is.
It's still tempting to think about the distribution of $\bar X$ as one that looks more and more like normal, i.e. taking a shape of the normal distribution. However I wouldn't use the term converges, or even approaches, as they are loaded in mathematics. You'll run into people who will be confused with this language for they will expect to see the non changing right hand side.