3
$\begingroup$

I was just wondering if there was a simple probabilistic argument for why a Poisson random variable with parameter $\lambda_1$ plus a Poisson random variable with parameter $\lambda_2$ (both independent) is a Poisson random variable with the sum of the parameters as a parameter.

I know one exists for binomial but can't think of one for Poisson.

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ If $\lambda_1\neq \lambda_2$, they're not iid, and if $\lambda_1=\lambda_2$, why distinguish them? But you can argue directly from the Poisson process itself (without needing the two Poissons to be identically distributed) $\endgroup$
    – Glen_b
    Commented Nov 4, 2015 at 1:25
  • $\begingroup$ Really? What about the poisson process allows for the sums to be poisson processes? $\endgroup$
    – conv3d
    Commented Nov 4, 2015 at 2:10
  • 1
    $\begingroup$ Because the superposition of the two processes (which count of events is the sum of the two Poisson counts) satisfies all the original conditions for the Poisson process. $\endgroup$
    – Glen_b
    Commented Nov 4, 2015 at 2:14

2 Answers 2

Reset to default
7
$\begingroup$

As Glen_b noted in comments, think of a Poisson variable as counting the number of events for a Poisson process of intensity $\alpha$ in a fixed window of size $w$: the Poisson parameter for this variable is $\alpha w$.

Suppose now there are two independent Poisson processes separately running in the same window, one of intensity $\alpha_1$ with $\alpha_1 w = \lambda_1$ and the other with intensity $\alpha_2$ with $\alpha_2 w = \lambda_2.$

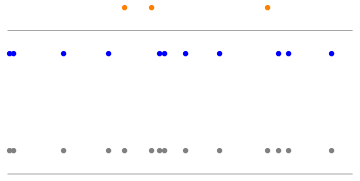
Figure

This figure shows realizations of two independent Poisson processes on the line within the window displayed as a gray line segment: one is shown as the orange points and the other as blue points. Their counts are realizations of two Poisson variables. By ignoring the colors, as shown at the bottom, we obtain a realization of a Poisson process whose intensity is the sum of the original intensities. The count is the sum of the counts.

The sum of these counts clearly is a Poisson process of intensity $\alpha_1 + \alpha_2$ because it trivially satisfies all the requirements of Poisson process (the count is proportional to the size of the window, events in non-overlapping windows are independent, and the chance of a count exceeding $1$ grows vanishingly small as the window size shrinks to zero). Therefore the sum of counts is a Poisson random variable with parameter $(\alpha_1+\alpha_2)w = \lambda_1+\lambda_2,$ QED.

Improve this answer
$\endgroup$
2
$\begingroup$

If you're satisfied with the fact that binomials sum to binomials when their parameter $p$ is the same, then you can view Poisson distributions as binomials with rare success that scales like $p\approx c/n$. This correspondence is exact in the limit so two Poissons sum to another Poisson immediately follows from the binomial case. In the case where the binomials have different probabilities $p_1,p_2$, they sum to a binomial with an adjusted parameter, whose adjustment approaches $p_1+p_2$ as both $p$'s get very small.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.