How to test whether a time series of measurements have converged to an equilibrium

Question

I have a time-series of data that looks like this (as a couple of examples):

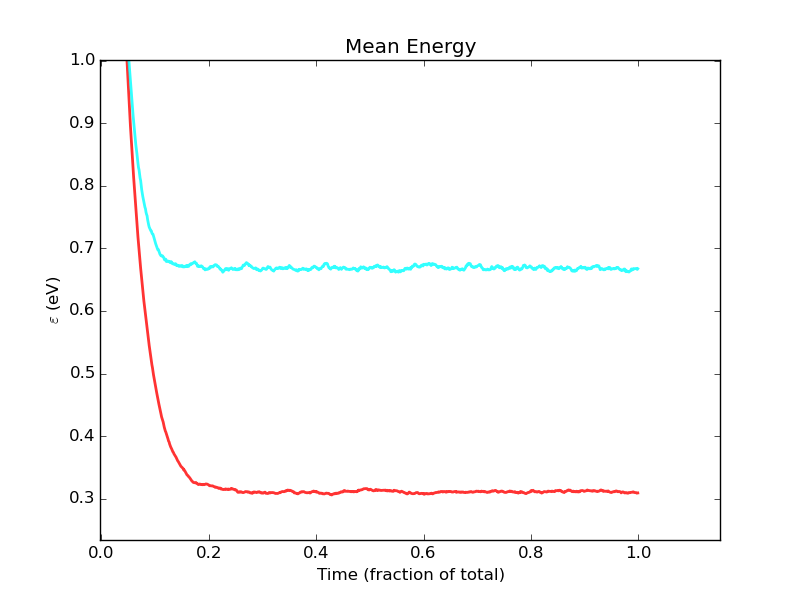
This mean energy is the mean over a number of Monte Carlo test particles. The number of particles vs. time is not necessarily constant.

It's clear that both series appear to converge on particular equilibrium values, and by eye-balling it, I'd say that it happens at around t = 0.3 for the cyan line and t = 0.4 for the red line (to be conservative).

My rough definition of reaching equilibrium is that once reached, all subsequent samples lie close to the equilibrium. Defining 'close' is tricky, though.

How can I determine the equilibrium time statistically? There are several problems that I've encountered in my naive home-grown attempts:

• The initial energy may be lower or higher than the equilibrium, and may not move monotonically - there can be a great deal of variation before it settles into equilibrium.
• The variance at later times may abruptly get much larger, as the number of samples used to calculate the mean drops off. I do track the number of samples though, so I can use that information too.
• The variance in the equilibrium may be so large that I can't tell whether the equilibrium is reached by eye-balling it. I'd like the test or algorithm to fail gracefully in such a case, and tell me that there is no clear solution.

Answer 1

Calculate the root mean square deviation in a sliding window over the course of a time series. Set a threshold to define "Convergence". Stop when the root mean square value falls below the threshold. i.e.

$$WindowRMSD_j=\sqrt{\frac{\sum_{i=1}^n(x_i-\bar{x})^2 }{n-1}}$$

where $n$ is the window size, $j$ is the current window, $x_i$ is the $i$th value in the window, and $\bar{x}$ is the mean of the values in the window. The first element in the window should begin with the index $i=0$.

As the time-series approaches convergence, the WindowRMSD will approach zero if the values converge.