1
$\begingroup$

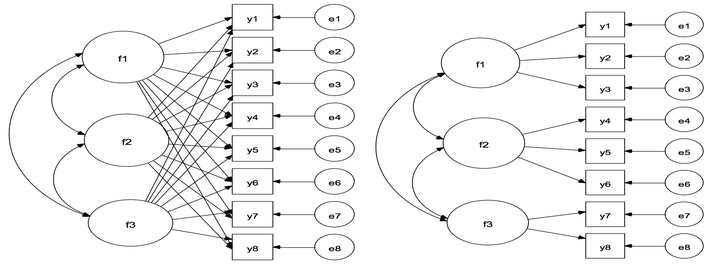
I have in mind figures like the following, which purport to explain the difference between EFA (left) and a 'standard' CFA (right). I guess in this picture one loading per factor should be fixed, and the authors left that off for the sake of simplicity.

EFA left CFA right

But will I get the same results if I take the same data and run
1. An obliquely rotated EFA
2. A CFA with every variable loading on every factor (i.e. like the one on the left).

Assume that the CFA

  1. Has the same estimation method as the EFA.

  2. Calls for the same number of factors as the EFA.

  3. Allows every observed variable to load on every factor.

  4. Allows all the factors to correlate.

Will the estimates of the standardized loadings and of the factor correlations still be the same? What about the unstandardized loadings? Will anything be different?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ It is a bit vague what you are asking. CFA and EFA have quite different goals, they can hardly compare. EFA finds (extracts) factors for you. In CFA, you construct factors by setting some their properties and constraining other. CFA is actually a form of SEM or path analysis; it is not actually FA, not a version of "factor analysis" per se. $\endgroup$
    – ttnphns
    Commented Apr 8, 2016 at 7:49
  • $\begingroup$ I edited the question to make it clear that I'm asking under what conditions an EFA algorithm within a statistics program would produce the same loadings as a CFA algorithm. $\endgroup$
    – user1205901 - Слава Україні
    Commented Apr 8, 2016 at 9:25

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.