3
$\begingroup$

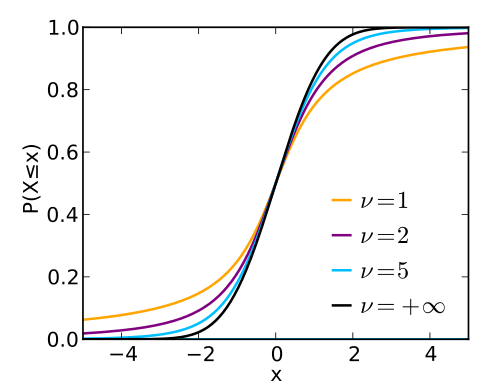
I have two non-central t distributions with CDF given by $t_{n,a}$ and $t_{m,a}$ with $n>m$ degrees of freedom respectively and the same non-centrality parameter $a$.

The question is: for which values of $x$ the inverse CDF of the first is greater than the inverse CDF of the second?

I.e. for which values of $x$ is $t^{-1}_{n,a}(x)>t^{-1}_{m,a}(x)$ ?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ If a numerical answer is enough, you can find it yourself inR $\endgroup$
    – kjetil b halvorsen ♦
    Commented Apr 28, 2016 at 8:09
  • $\begingroup$ I know, but I'd like to have a theoretical answer, if it's possible. $\endgroup$
    – xanz
    Commented Apr 28, 2016 at 8:26
  • $\begingroup$ @Xi'an the limit value for $x$ is that value for which the $t_{n,a}(x)=t_{m,a}(x)=0$? I.e. the answer is when $x<t_{n,a}(0)$? $\endgroup$
    – xanz
    Commented Apr 28, 2016 at 8:43

1 Answer 1

Reset to default
2
$\begingroup$

Since the non-centrality parameter is irrelevant for this question, I take $a=0$.

Here is a picture of several cdfs of Student's $t$-distributions:enter image description here

And an even better image from Wikipedia: enter image description here

As the $t_n$ distribution has fatter tails than the $t_m$ distribution when $n<m$, hence the former is be less concentrated around its mean/mode/median zero than the latter. This means the cdf of $t_n$ will be larger than the cdf of $t_m$ for large enough negative values of $x$ and smaller for large enough positive values of $x$. As you can see on the picture, all cdf's cross at zero (the slope of the cdf at zero $F(0;n)$ is the value of the pdf at zero $f(0;n)$ which is increasing with $n$).

Improve this answer
$\endgroup$
4
  • $\begingroup$ But in the case of a non-central t-distribution, the shift due to the non-centrality parameter does it change things? $\endgroup$
    – xanz
    Commented Apr 28, 2016 at 9:06
  • $\begingroup$ The answer is right, I'm just answering my own previous comment: no, it doesn't. The CDF of two noncentral t-distributions evaluated in 0 is always equal to $\Phi(-a)$ if the noncentrality parameter $a$ is the same (check the CDF expression on wikipedia), regardless of the dofs. And if $n>m$ the tails of $m$ are fatter than the tails of $n$, thus if $x<\Phi(-a)$ then the requirement in the question is satisfied. Thank you! $\endgroup$
    – xanz
    Commented Apr 28, 2016 at 9:15
  • 1
    $\begingroup$ @xanz: your answer only mentioned the same non-centrality parameter, which is indeed irrelevant for the tail comparison. If you had different non-centrality parameters then the cdfs could cross more than once. $\endgroup$
    – Xi'an
    Commented Apr 28, 2016 at 9:31
  • $\begingroup$ Yeah, now I know. At first it wasn't clear to me that the noncentrality parameter is irrelevant for the number of crossings, but now I've figured it out. Thank you. $\endgroup$
    – xanz
    Commented Apr 28, 2016 at 10:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.