I am using Gibbs sampling in the MCMC estimation of a stochastic volatility model. One of the posterior distributions is an Inverse Gamma distribution.
I was struggling with the sampling procedure or to be precise with the link to the Gamma distribution. Is it true that to get $Y \sim IG(alpha,beta)$, I have to sample $X \sim Gamma(\alpha,1/\beta)$ and take $ Y = 1/X$? This is also inline with posts on mathworks.
However, I also read it up on wolfram and in my opinion they contradict themselves in the article (https://reference.wolfram.com/language/ref/InverseGammaDistribution.html).
From the paragraph Background&Context (which deals with the generalized gamma distribution though):
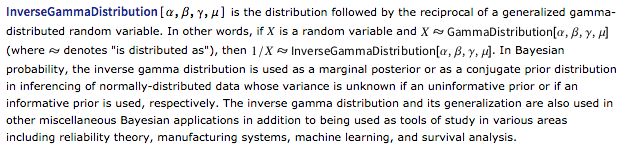
vs. the description from Details (specifically the fourth point):
Does this discrepancy arise due to the difference between the inverse gamma and the generalized inverse gamma?

