Does q-function remain the inverse of CDF for any type of distribution? [closed]

Question

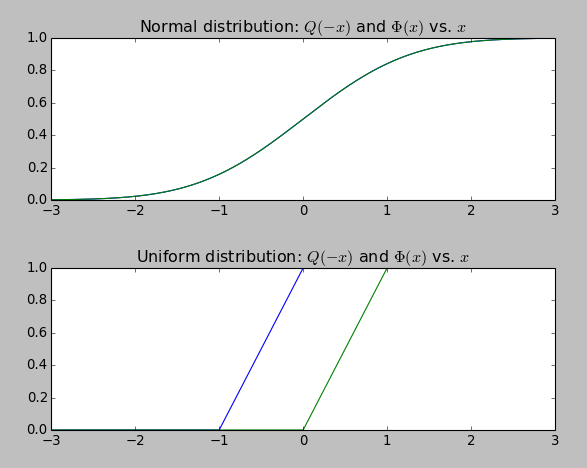
I read the the Quantile Function commonly known as q-function is the inverse of the CDF for a normal distribution.

$Q(x) = 1 - Q(-x) = 1 - \Phi(x)\,\!,$

where Φ(x) is the cumulative distribution function of the normal Gaussian distribution.

Does this apply to any kind of distribution, or only to the normal distribution?

Answer 1

No.

The expression $Q(-x)=\Phi(x)$ only holds when $\Phi$ (and thus also $Q$) is symmetric around $0$, like for the Gaussian distribution.

One example where this is not the case is the uniform distribution:

PS. Just to be clear: The $Q$ function you refer to in your link is not the same as the quantile function.