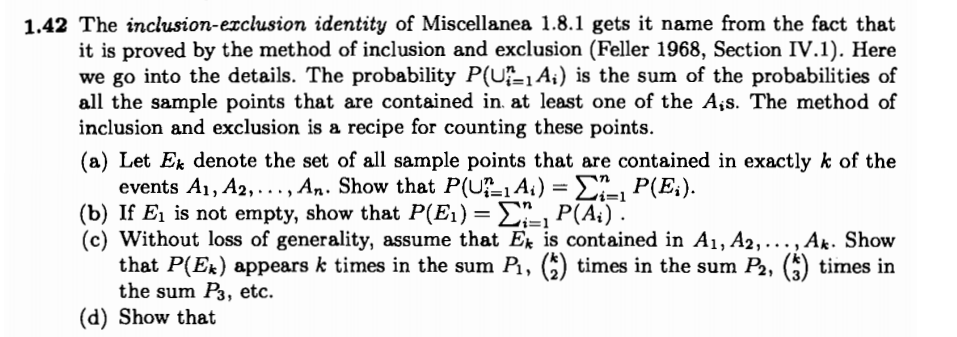
I am currently self-studying with Casella & Berger and there is a problem that has me stumped. Please note I am NOT asking for a solution, I just need clarification on the terms used.
Here is the exercise in question:
The way I understand the definition of the set Ek, it is not generally true that P(E1) = P(A1) + ... + P(An).
To illustrate, with A1 = {1,2} and A2 = {1,2,3}, E1 would denote elements contained in exactly one of A1 and A2, namely E1 = {3}. The statement in problem (b) would therefore be equivalent to P({3}) = P({1,2}) + P({1,2,3}).