1
$\begingroup$

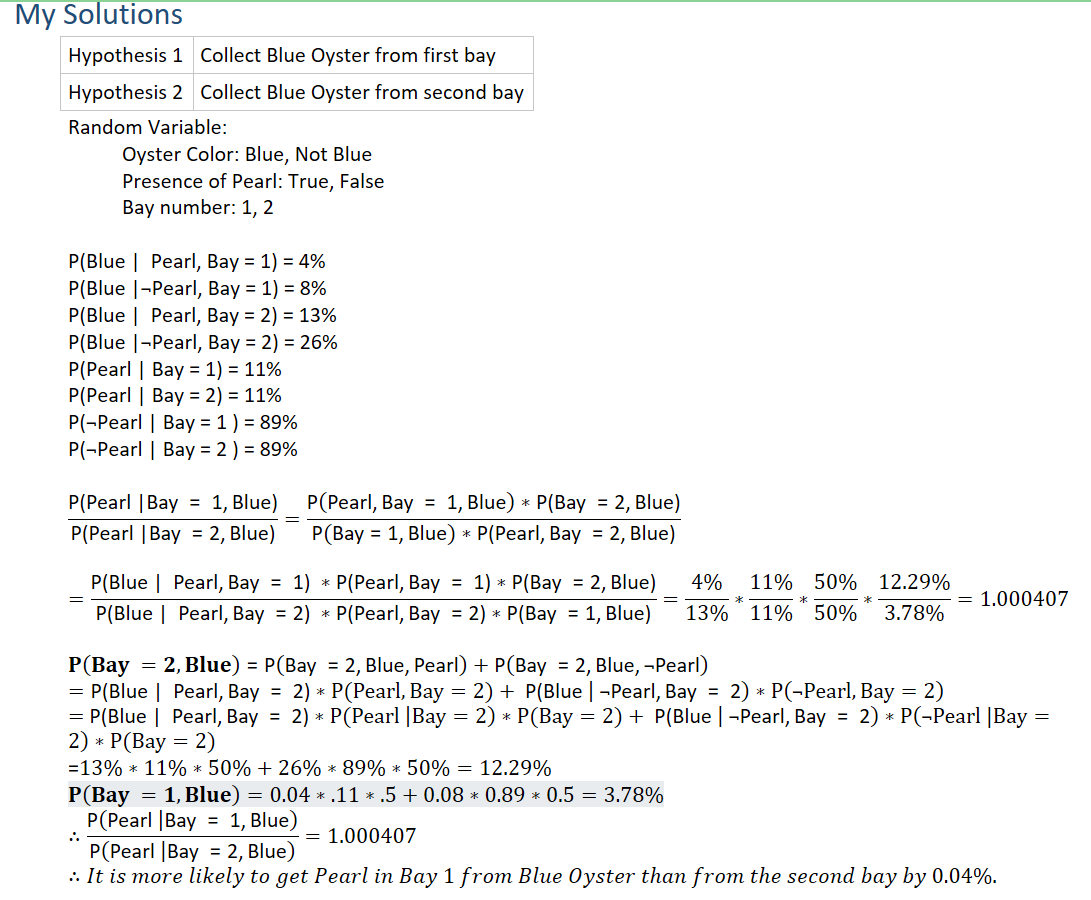
Problem: You're collecting exotic oysters, and there are two different bays from which you could harvest oysters. • In both bays, 11% of the oysters contain valuable pearls and 89% are empty. • In the first bay, 4% of the pearl-containing oysters are blue, and 8% of the non-pearl-containing oysters are blue. • In the second bay, 13% of the pearl-containing oysters are blue, and 26% of the non-pearl-containing oysters are blue. Would you rather have a blue oyster from the first bay or the second bay?

I have solved the problem, but I am not sure if its correct. Please comment if possible!

My Solution: enter image description here

Improve this question
$\endgroup$
2
  • $\begingroup$ reopened from "off-topic" since this did already show an attempt. Do you have a specific question about your attempt you'd like addressed? $\endgroup$
    – Glen_b
    Commented May 15, 2017 at 1:55
  • $\begingroup$ I will say I'd have been inclined simply to calculate P(Pearl|Blue, Bay1) and P(Pearl|Blue, Bay2) directly and compare the two conditional probabilities to see which was larger, rather than take the ratio (not that there;s anything wrong with that idea) $\endgroup$
    – Glen_b
    Commented May 15, 2017 at 2:09

1 Answer 1

Reset to default
1
$\begingroup$

You appear to have prematurely rounded at one point in each calculation and then discovered a tiny difference.

  1. How do you know that apparent difference isn't simply rounding error?

  2. Consider writing your answers as exact fractions.

  3. (Alternatively) Both the calculations are special cases of the following problem:

    11% of the oysters contain valuable pearls and 89% are empty. In a bay, a proportion $q$ of the pearl-containing oysters are blue, and $2q$ of the non-pearl-containing oysters are blue.

    Find an expression for P(Pearl|Blue) for this problem. Divide numerator and denominator by $q$. What do you notice?

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.