I'm going through Schumacker's Learning Statistics Using R. I've come across something that's not making too much sense to me, so I'm wondering if this is a mistake or if I'm just really thick.
On page 46, the textbook reads
If the dice are unbiased , 36 outcomes are possible (6 x 6). Each outcome (S) is a sum of the number on two dice. The probability of any sum, S, can be calculated by the formula: P(S) = (S/36). For example, P(3) = 1/12; the number of times two dice sum to 3 divided by the total number of outcomes, 36, which has a probability of .0833
Now there's my first problem. That formula doesn't seem right. There are only 2 possibilities of getting 3, rolling a 1 on the first die and a 2 on the second or rolling a 2 on the first die and a 1 on the second, which makes the probability 2/36 = 1/18 = .0556 NOT .0833. This is the bit where, I could be the thick one, because, to me, the formula seems faulty. It doesn't seem to be the sum, more like the count. .0833 would not work with 3, but it would work with 4, which has 3 combinations that would result in 4.
So that's one, but then I have an issue with the probabilities the text asks to input into R.
It gives the following
Probs = c(.0556,.0833,.1111,.1389,.1667,.1667,.1389,.1111,.0833,.0556,.0278)
This doesn't seem right at all. I'm not sure what the author is trying to say. Is he, maybe, showing something other than what I think he's trying to show? 2 and 12 should have the same probabilities; according to the text, rolling a 2 has a higher probability than 12. Also 7 should have the highest probability, given that there are 6 combinations that will yield 7, and there are only 5 combinations that result in a 6, so how can the probabilities of rolling a 6 and 7 be the same?
Shouldn't the probabilities read as the following instead
Probs = c(.0278,.0556,.0833,.1111,.1389,.1667,.1389,.1111,.0833,.0556,.0278)
This is bringing me a great deal of tension. Any and all help in explaining this to me, if I don't get it, will be greatly appreciated.


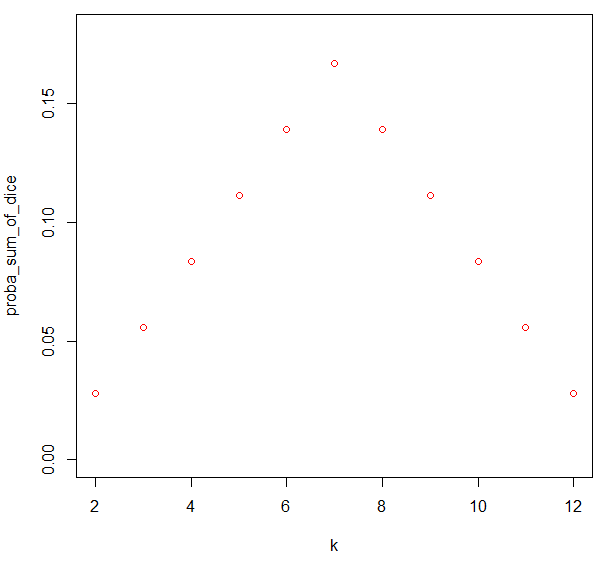
P(S) = S/36is plain wrong and rather bizarre! The 2nd mistake could be regarded as an annoying but trivial typo; it's obviouslyProbs = c(1,2,3,4,5,6,5,4,3,2,1) / 36which comes to the numbers you wrote. $\endgroup$