Is this a conditional distribution or joint, or none?

Question

I have collected some data on two variables (see Table below). Variable #1 is called "p-value" and has 4 categorical levels. Variable #2 is called "Bayes Factor" and has 7 categorical level.

Question

Is what I have a contingency table? In other words, does the table I'm showing below indicate the conditional frequency distribution of one variable given the other or the joint frequency distribution of the two variables together? If not what the table below is called, statistically speaking?

Answer 1

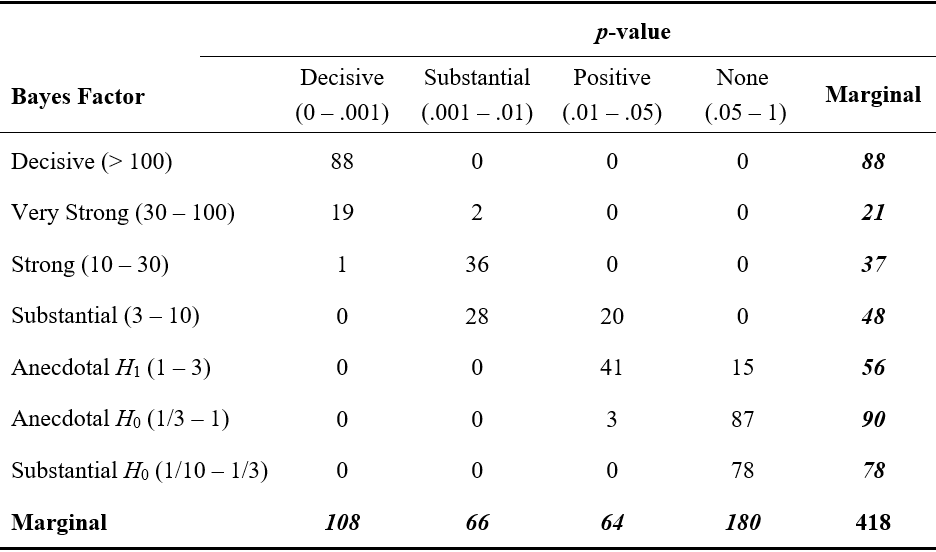
General

This is a contingency table. It shows you for every possible combination of Bayes Factor and p-value, how many time it occurred in your data. The entries in your table are counts (AKA frequencies). The bold-faced numbers in the margins of the table are the row and column totals.

2 Examples

For example, you can read from the table that:

1. there are 418 data points.
2. 108 of those have p-value of Decisive.
3. That correspond to a probability of $108/418 = 0.258$

Another example: 28 observations have a Bayes' Factor of Substantial while also having Substantial for the p-value. That corresponds to a probability $28/418 = 0.067$

Conditional frequency distribution

Taking it a step further. You can also only focus on 1 row or column of the table. By doing this, you can make statements such as: Conditional on a positive p-value, there is a probability of 20/61 = 0.328 that the observation has a value of Substantial for the Bayes' Factor. By focusing on 1 row or column, you are focusing on marginal distributions, i.e. conditional distributions.

Update

In response to your comment, I will deal with the two follow up questions you posed:

1. What is P(p.value = Positive | Bayes Factor = Substantial)?

Since we condition on Bayes Factor = Substantial, we will ignore all rows, except Bayes Factor = Substantial and take that row's total as our total (The blue circled 48). Then we look for the column where p-value = Substantial and see there are 28 (blue circled 28) observations: P(p.value = Positive | Bayes Factor = Substantial) = 28/48

2. What is P(Bayes Factor = Substantial | p.value = Positive)?

With the same logic, we should now look at the p-value = Positive column and ignore all the rest. In that column, search for the cell where Bayes Factor = Substantial and divide the two:

P(Bayes Factor = Substantial | p.value = Positive) = 64/20