1
$\begingroup$

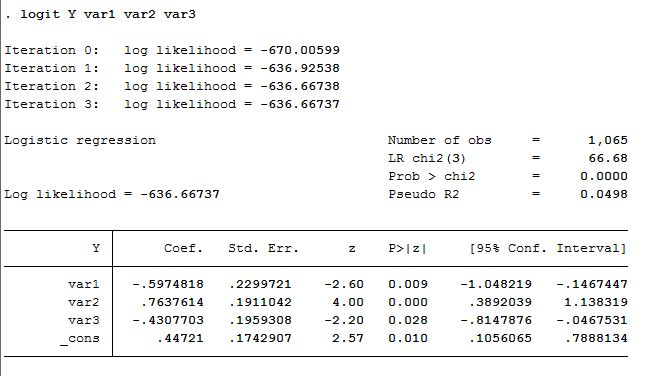
I have a logistic regression model: logit(Y)= b0+b1+b2+b3. All my variables are dummy variables.

logistic regression without interaction term

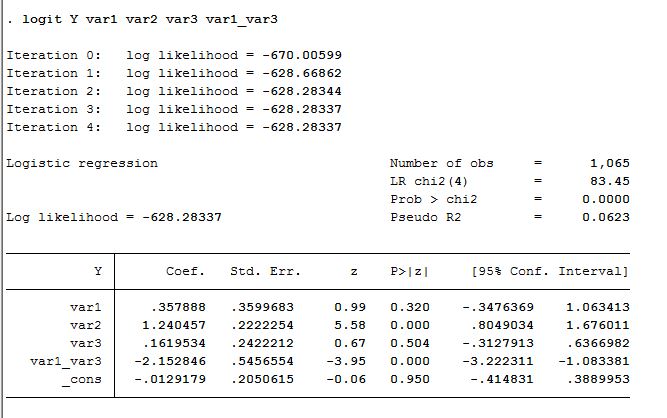
I want to add the interaction term to the model: logit(Y)= b0+b1+b2+b3+b1*b3. Please, find the model with interaction term below. I would like to know how the interpretation of the variables var1, var2, and var3 changes when the interaction term is added to the model. For instance, is the coefficient of var2 1.24, when var1=0 and var3=0. What is the reference category for the interaction term? Is it var1=0, var2=0, var3=0? Is the constant when var1=0, var2=0, var3=0?

logistic regression with interaction term

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

The interpretation of the coefficient for var2 stays the same: the difference in log odds of the outcome between being in level 0 and level 1 of var2, holding constant var1 and var3 (and, therefore, var1_var3). The interpretation of the coefficient for var1 is now the difference in log odds of the outcome between levels 0 and 1 of var1 when var3 is equal to 0 and var2 is held constant. Replace var1 and var3 in the above explanation for the interpretation of the coefficient of var3.

The coefficient for the interaction is the difference in the effect of var1 between levels 0 and 1 of var3. So, the effect of moving from level 0 to level 1 of var1 when var3 is equal to 1 is 0.357888 - 2.152846 (whereas, the effect of moving from level 0 to level 1 of var1 when var3 is equal to 0 is just 0.357888).

Improve this answer
$\endgroup$
2
  • $\begingroup$ Note that the interpretation of the interaction term might depend on how the model is formulated. What you described is the case for treatment contrasts with the lowest level of each categorical/dummy variable taken as the reference, the default in R. Some statistical software, however, might take the highest level of a dummy variable as the reference, or use different contrasts, which might alter the details of interpretation of the interaction term. $\endgroup$
    – EdM
    Commented Sep 11, 2017 at 18:17
  • $\begingroup$ @EdM, true, although based on OP's Stata output I think what I've provided is accurate. $\endgroup$
    – Noah
    Commented Sep 11, 2017 at 21:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.