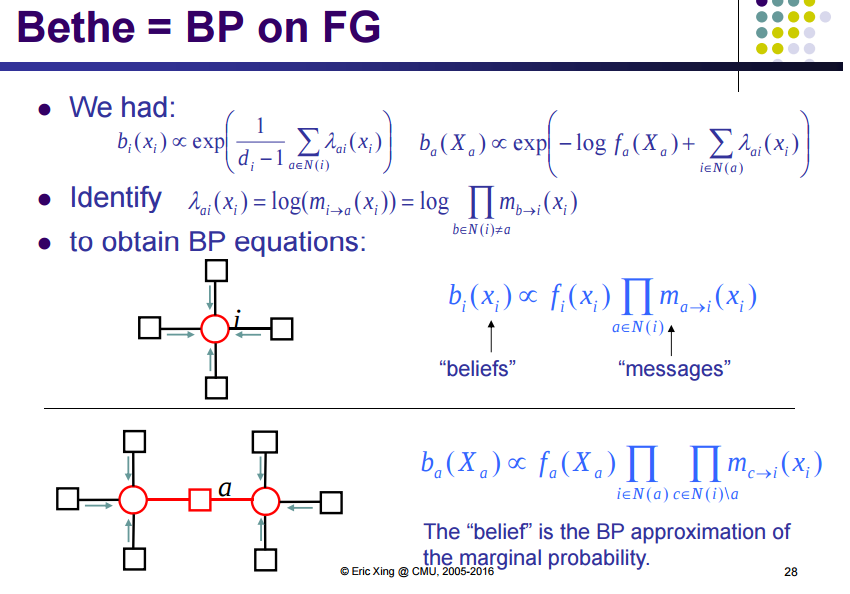
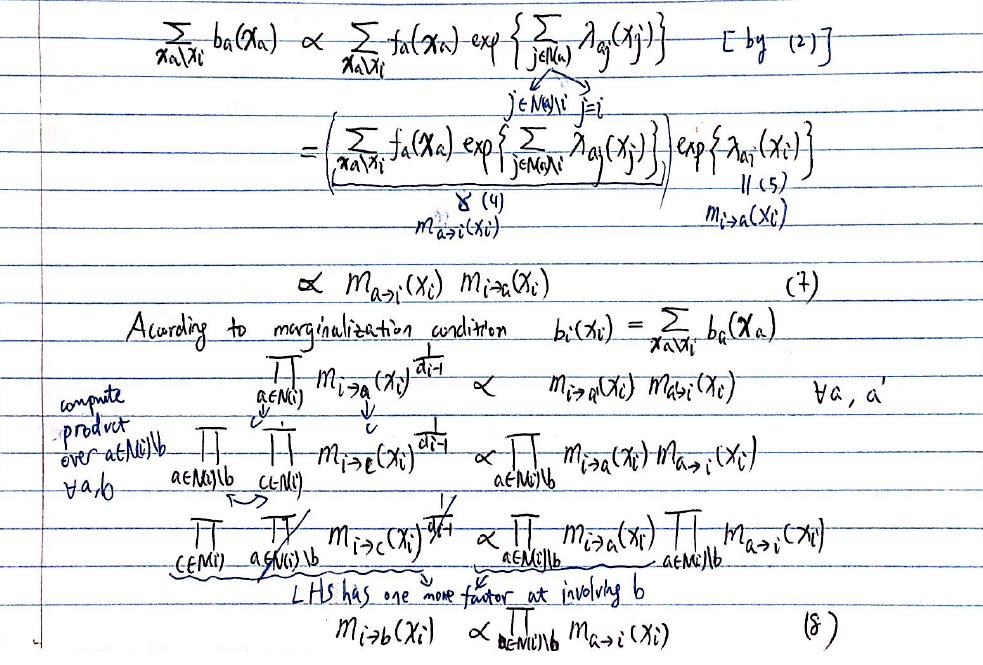
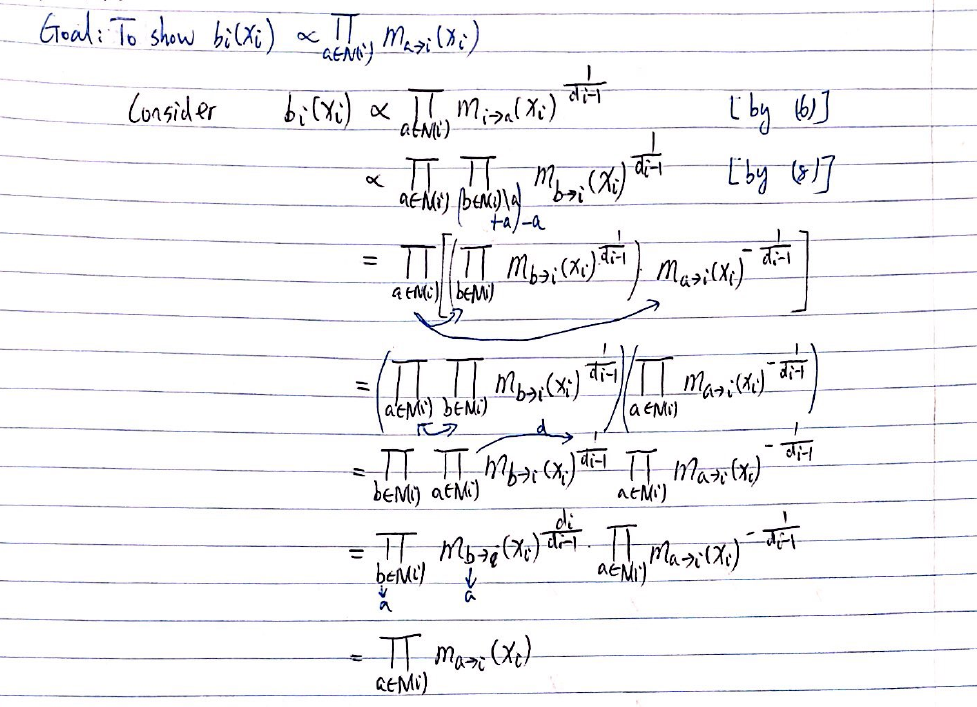
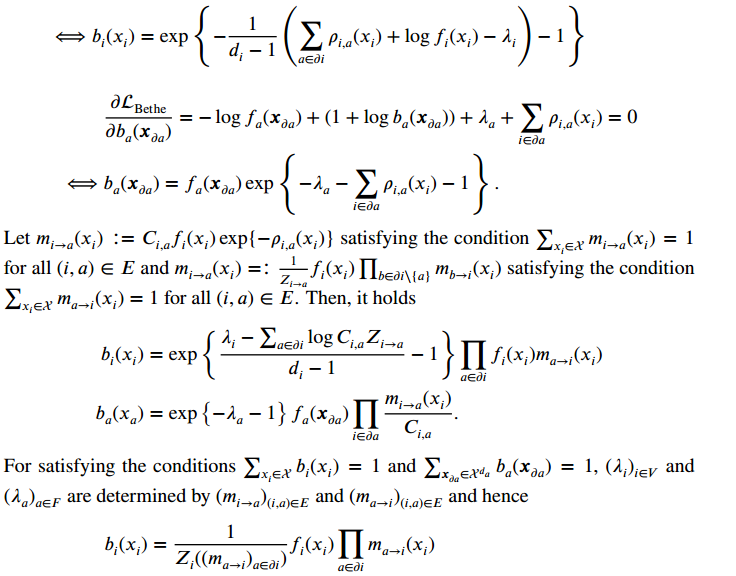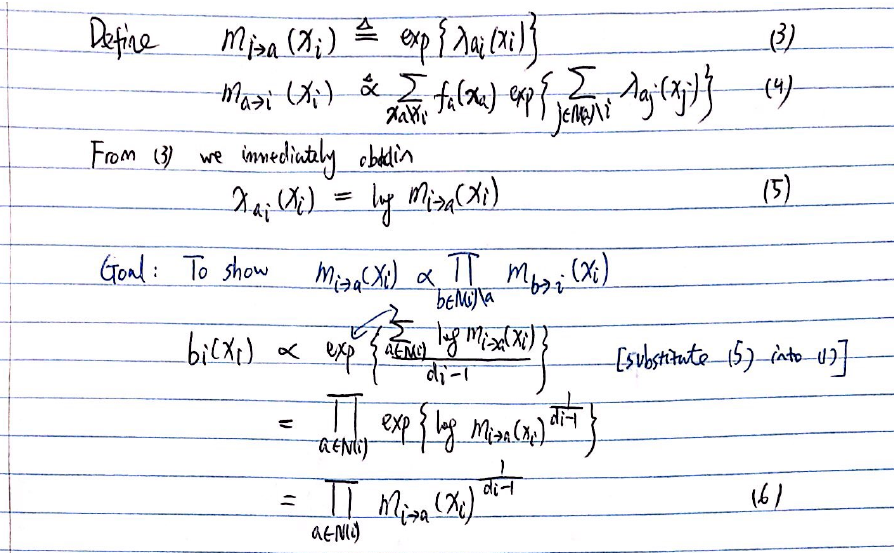I am confused at computing Bethe approximation for factor graphs in here. It generalizes Bethe approxmiation in a pairwise case. However, I am wondering why (75) goes to (78) with (76):
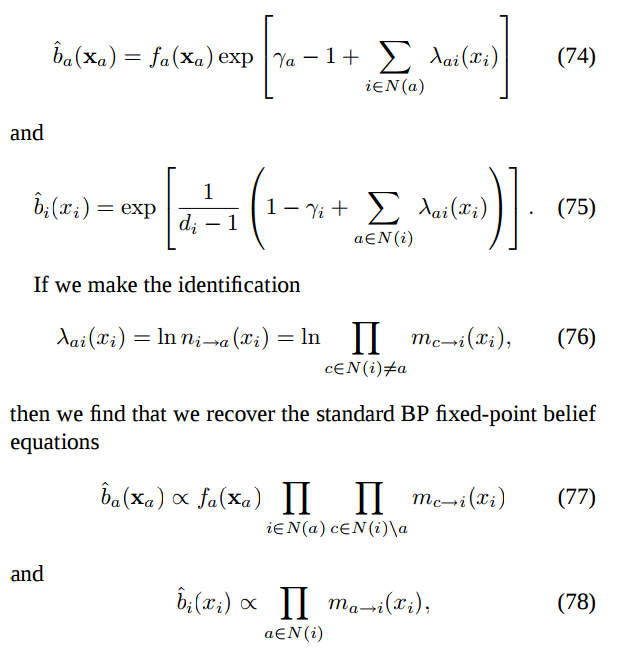
We can verify that
$$\hat b_i(x_i)\propto \exp\left[(d_i-1)^{-1}\sum_{a\in N_i}\lambda_{ai}(x_i)\right]$$
$$\begin{align}\hat b_i(x_i)&\propto \exp\left[(d_i-1)^{-1}\sum_{a\in N_i}\lambda_{ai}(x_i)\right]\\ &=\prod_{a\in N(i)}\exp\left[(d_i-1)^{-1}\lambda_{ai}(x_i)\right] \end{align}$$
Since (76) involves $m_{\text{fact}\rightarrow\text{singleton}}$, it's tempting to use the second equation in (76), and thus we have
$$\begin{align}\hat b_i(x_i)&\propto \prod_{a\in N_i}\exp\left[\log \prod_{c\in N_i\backslash a}m_{c\rightarrow i}(x_i)^{\frac1{d_i-1}}\right] \end{align} $$
Then everything is messed up. I do not see why after canceling $\exp$ and $\log$ we obtain $m_{a\rightarrow i}(x_i)$.
There are several references where $m_{a\rightarrow i}$ all comes out mysteriously. I am looking for help in this derivation.
Other refs:
- p. 28 here:
- p. 24 here: