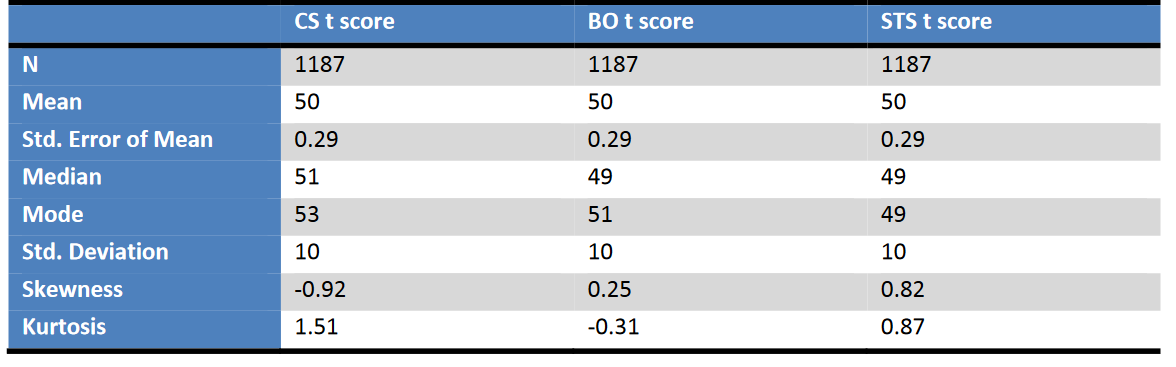
I have the t score data in the image below for three datasets. Is it possible to calculate the raw score mean for each dataset using this data? I know that the raw score means are somewhere between 23 and 41
$\begingroup$
$\endgroup$
4
-
1$\begingroup$ Could you please tell us what a "raw score mean" is? Your table shows three means, all equal to 50, and as far as I can tell that would be a "raw score mean." Maybe you need to explain further what this table is trying to show. $\endgroup$– whuber ♦Commented Sep 6, 2018 at 20:54
-
$\begingroup$ The table has the mean t score, which was converted from the raw score. I'm wondering if I can get back to the raw score using the data that is in the table $\endgroup$– Michael CCommented Sep 6, 2018 at 21:02
-
$\begingroup$ Could you perhaps explain what a "t score" is then? I had thought it might be something like a t statistic, but then it would be very unusual to have a dataset in which all mean t scores are close to 50. $\endgroup$– whuber ♦Commented Sep 6, 2018 at 21:08
-
$\begingroup$ (1) Notice that SE Mean $0.29 = SD/\sqrt{n} = 10/\sqrt{1187}.$ (2) In the usual language of 'raw' and 'standard' scores: if $X$ is raw (observed) score, then standard score is $Z = \frac{X - \mu}{\sigma},$ were $\mu$ and $\sigma$ are population mean and SD, respectively. So if $X \sim NORM(\mu,\sigma),$ then $Z \sim NORM(0,1),$ standard normal. I am not familiar with your use of "raw score" and "t-score." Helpful if you can find formulas. $\endgroup$– BruceETCommented Sep 7, 2018 at 0:17
Add a comment
|
