In a real-valued multivariate case, is there a way to uniformly sample the points from the surface where the Mahalanobis distance from the mean of the is a constant?
EDIT: This just boils down to sampling points uniformly from the surface of a hyper-ellipsoid that satisfies the equation,
$$(x-\mu)^T \Sigma^{-1}(x-\mu) = d^2.$$
To be more precise, by "uniformly", I mean sample such that each area element $dA$ of the hyper-surface contains the same probability mass.
When the different ellipsoid axes are not too much different then it is feasible to use rejection sampling (with large differences you reject a lot making it less feasible)
2D example
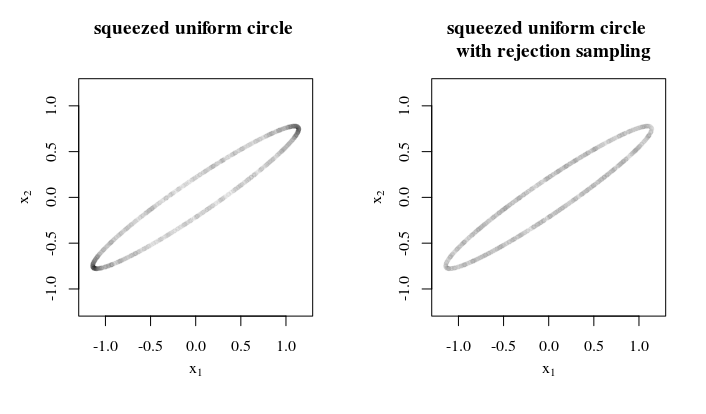
set.seed(1)
#some matrix to transform n-sphere (in this case 2x2)
m <- matrix(c(1, 0.55, 0.55, 0.55), 2)
# sample multinomial with identity covariance matrix
x <- cbind(rnorm(3000, 0, 1), rnorm(3000, 0, 1))
l1 <- sqrt(x[,1]^2 + x[,2]^2)
# perpendicular vector
per <- cbind(x[,2], -x[,1])
# transform x
x <- x %*% m
# transform perpendicular vector (to see how the area transforms)
per2 <- per %*% m
# get onto unit-"sphere"/ellipsoid
x <- x/l1
# this is how the area contracted
contract <- sqrt(per2[,1]^2 + per2[,2]^2) / sqrt(per[,1]^2 + per[,2]^2)
# then this is how we should choose to reject samples
p <- contract/max(contract)
# rejecting
choose <- which( rbinom(n=length(p), size=1, p=p) == 1)
#plotting
plot(x[1:length(choose), 1], x[1:length(choose), 2],
xlim=c(-1.2, 1.2), ylim=c(-1.2, 1.2),
xlab = expression(x[1]), ylab = expression(x[2]),
bg=rgb(0, 0, 0, 0.01), cex=0.6, pch=21, col=rgb(0, 0, 0, 0.01))
title("squeezed uniform circle \n ")
#plotting
plot(x[choose,1], x[choose,2],
xlim=c(-1.2, 1.2), ylim=c(-1.2, 1.2),
xlab = expression(x[1]), ylab = expression(x[2]),
bg=rgb(0, 0, 0, 0.01), cex=0.6, pch=21, col=rgb(0, 0, 0, 0.01))
title("squeezed uniform circle \n with rejection sampling")
