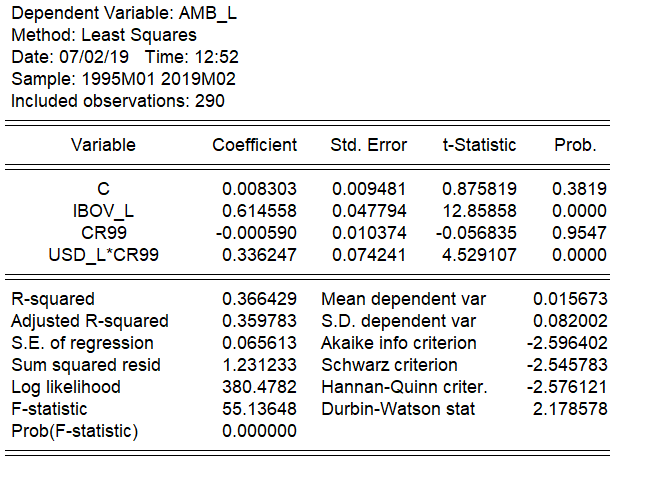
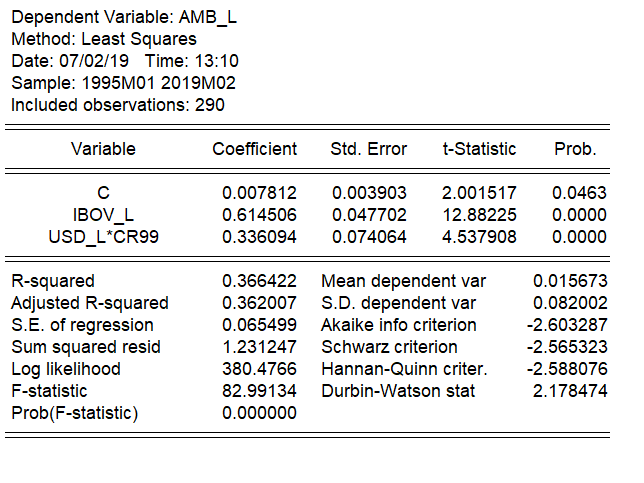
I have the following model based on the financial returns of a company as a dependent variable of a stock market index, and a dummy variable interacting with USD exchange rates to my currency. The dependent variable and the stock index returns are in log.
The dummy variable is basically 0 before my country switched our exchange rates to floating in 1999 (exchange rates used to be fixed by the government) and 1 afterwards.
Where IBOV_L is the stock index and CR99 is the dummy variable.
My question is: can I remove the "standalone" dummy variable and leave only the interaction in my model? Because the p-value is very insignificant.
Also, I can't run the chow stability test if I don't remove it, I get an error saying something about "singular matrix". Is it ok to use a dummy variable interacting with the exchange rates in the model but not the exchange rates and the dummy by themselves?
In other words, is the model below ok? Does it make sense?
This is probably a silly question but I'm just beginning studying this.