Geometric distribution with multiple trials

Question

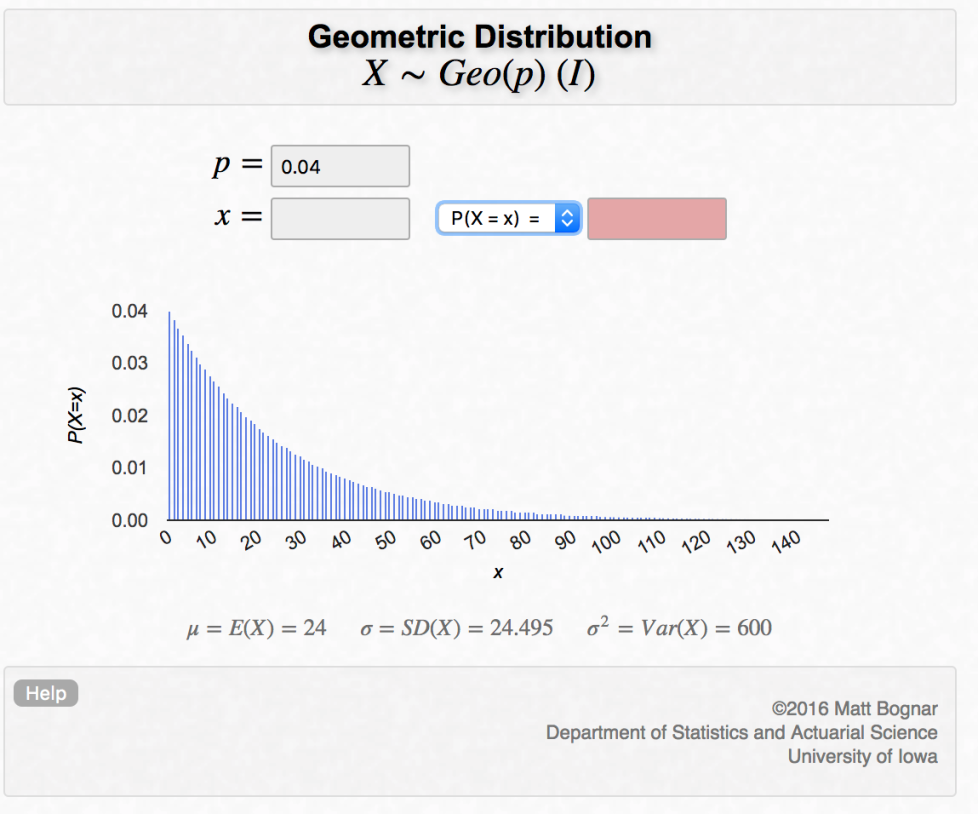
I was looking into geometric distributions to find the probability of the first success of some random variable X. So if p = 0.04, the geometric distribution looks something like this:

I understand the graph mathematically from the probability formula, but it seems kind of unintuitive for the chance of first success being x to keep going down, since I wouldn't expect getting a success on the first try to have the highest probability with p = 0.04.

So if I run an experiment with a random number generator with chance of success = 0.04, and record the trial in which I get my first success and plot it on a graph, would it be expected to look something like the above distribution, where getting a success on the first try would be the most common event?

Answer 1

As whuber notes, to succeed on the second trial, you must fail and then succeed. To succeed on the first trial you need only succeed -- and both successes happen with the same probability, so success on the second trial is less probable than the first.

Yes, if you simulate this process you should see something like the probability function you have shown (plus some variation due to random noise). The larger the simulation, the more the sample proportions should reflect the population proportions.