Correct or Not? Probability and Geometry [closed]

Question

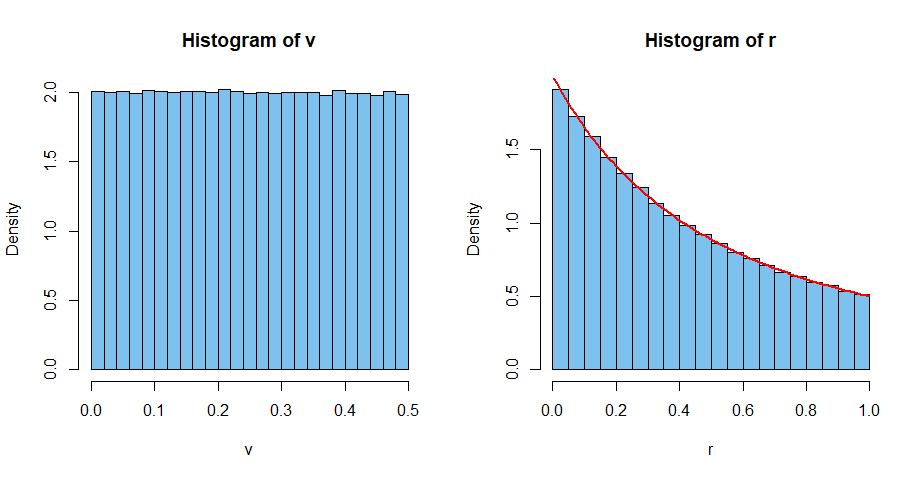
A stick of length $1$ is broken into two pieces of length $Y$ and $1−Y$ respectively, where $Y$ is uniformly distributed on $[0,1]$. Let $R$ be the ratio of the length of the shorter to the length of the longer piece.

Find the PDF $f_R(r)$ of $R$. Hint: What is the PDF of the length of the smaller piece? For $0<r<1$, For $f_R(r)$

I have $f_R(r)= {2\over(r+1)^2}$ or is the answer $f_R(r)= {1\over(r+1)^2}$

Also, $E[R]= ln(4)$ or is the answer, $E[R]= ln(2)−0.5$

Answer 1

Hints: Here's what I got by simulating the distribution in R. Your first density function seems to work, but neither of your expectations seem to be right. [Especially not $\ln(4) > 1;$ a typo maybe?] With a million iterations, one should expect about two place accuracy.

y = runif(10^6)
v = pmin(y, 1-y);  r = v/pmax(y, 1-y)
mean(r);  sd(r)
[1] 0.3856303
[1] 0.2794012
2*sd(r)/sqrt(10^6)
[1] 0.0005588025    # aprx 95% margin of simulation error

par(mfrow=c(1,2))
 hist(v, prob=T, col="skyblue2")
 hist(r, prob=T, col="skyblue2")
  curve(2/(x+1)^2, add=T, lwd=2, col="red")
par(mfrow=c(1,1))