@SergeyBushmanov points out that sometimes you need to give maximum likelihood estimation a hand by providing appropriate starting point and lower/upper bounds for the optimization algorithm to find the MLE.
Another way to give the likelihood a hand is to augment it with a (weakly) informative prior and do Bayesian estimation. This approach is most applicable if we have domain information to help us formulate a useful prior.
I'll illustrate one Bayesian solution to the $t$ distribution fitting problem in the setting where @kjetilbhalvorsen demonstrates that the MLE approach fails: sample size $n = 10$, location $\mu = 0$, scale $\sigma = 1$ and degrees of freedom $\nu = 2.5$. I'll use the brms package to do the Bayesian model fitting and two different priors for the degrees of freedom $\nu$:
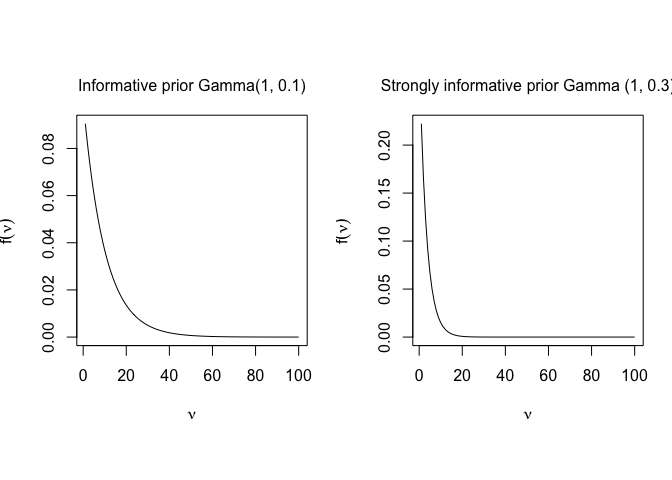
- strongly informative prior: $\nu \sim \operatorname{Gamma}(1, 0.3)$ and
- informative prior: $\nu \sim \operatorname{Gamma}(1, 0.1)$,
with the constraint $\nu > 1$ (otherwise the mean of the $t$ distribution is undefined.)
Both choices indicate there is high prior probability the degrees of freedom are less than 5: under the informative prior, $\operatorname{Pr}(\nu<5) = 0.33$ and under the strongly informative prior, $\operatorname{Pr}(\nu<5) = 0.7$. So both priors are quite "opinionated", which we would like to avoid in general. [The default brms prior on the degrees of freedom is $\operatorname{Gamma}(2, 0.1)$.] On the other hand, in general we would also probably collect more than $n = 10$ observations for a study where we know there is a lot of variability in the outcome.
And here is how to do Bayesian $t$ distribution fitting with brms::brm.
First we simulate a sample of size $n$ from $t(\mu=0,\sigma=1,\nu=2.5)$ where $\mu$ is the location, $\sigma$ is the scale and $\nu$ are the degrees of freedom.
n <- 10
mu <- 0
sigma <- 1
nu <- 2.5
x <- rt(n, nu) * sigma + mu
Then we fit an intercept-only $t$-family model.
fit.brm <- brm(
x ~ 1,
family = student,
data = data.frame(x),
prior = c(
prior(student_t(3, 0, 2.5), class = "Intercept"),
prior(student_t(3, 0, 2.5), class = "sigma"),
prior(gamma(1, 0.3), class = "nu")
)
)
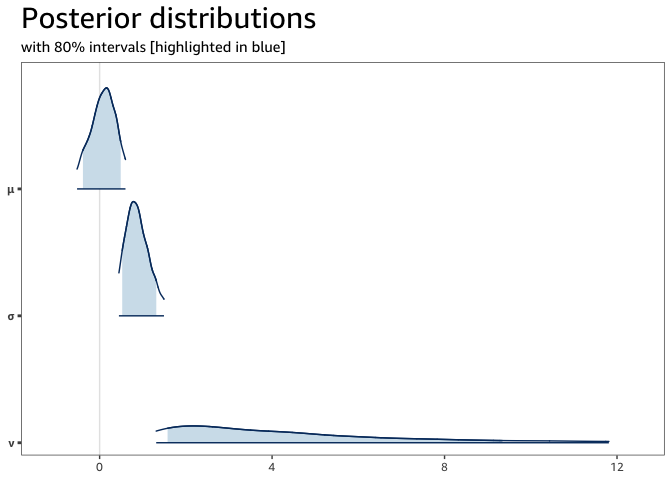
While the posterior distributions of the location $\mu$ and scale $\sigma$ are reasonably symmetric, the posterior of the degrees of freedom $\nu$ is very skewed. So I will use the posterior mode (rather than the posterior mean or the posterior median) to estimate the parameters.
round(
apply(posterior, 2, mode), 3
)
#> μ σ ν
#> 0.161 0.785 2.164
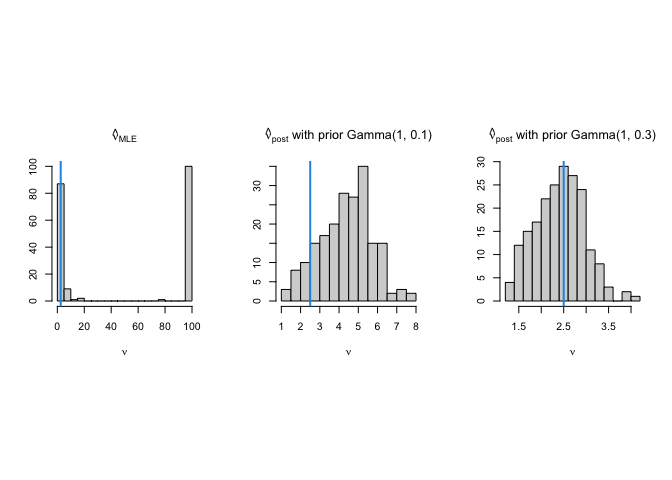
And finally, I repeat the three analyses (MLE, Bayesian with weakly informative prior, Bayesian with informative prior) 200 times. Each analysis estimates all three parameters (location $\mu$, scale $\sigma$ and degrees of freedom $\nu$) but below I plot histograms of the estimated degrees of freedom only. The true $\nu$ is 2.5.
Maximum likelihood estimation fails dramatically more than half of the time (100 is the upper bound for $\nu$ in the optimization). Bayesian estimation doesn't fail in any of the 200 simulations but the estimate is biased upwards unless the prior information indicates strongly that we expect a priori only a few degrees of freedom.