$\newcommand{\op}[1]{\operatorname{#1}}$
Let's use some abbreviations as follows:
\begin{align*}
\op{UTRS}&=\text{Upper Trunk Rotation Strength}\\
\op{I}&=\text{injuries}\\
\op{A}&=\text{Age}\\
\op{W}&=\text{Weight}\\
\op{PCS}&=\text{Posterior Chain Strength}\\
\op{GS}&=\text{General Strength}.
\end{align*}
Now the basic model under consideration is $\op{UTRS}\to\op{I}.$ As in, via causal diagrams, we are considering $\op{UTRS}$ as the cause of $\op{I}.$ Suppose, like the paper, we are considering age as a confounder; that would require something like this:
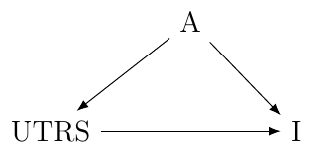
While I could certainly buy the arrow from $\op{A}\to\op{UTRS},$ it's not at all clear, given the age range in question of teenagers, why $\op{A}\to\op{I},$ unless you are considering that older teenagers are more experienced, and less likely to get an injury. That's a possibility. Similarly, we are right to question $\op{W}$ as a similar confounder. But here, the question would be, why does $\op{W}$ influence $\op{UTRS}?$ What would be the causal mechanism there?
The reason I bring up these questions is that, depending on your causal model, sometimes you should adjust or condition for variables because they confound the results - that is, they produce a backdoor path such as in my diagram above, where $\op{UTRS}\leftarrow\op{A}\to\op{I}$ is a backdoor path. That is a true confounder. But what if, e.g., with weight, the arrow goes the other way? Like this:

If this is the case, and I could imagine a scenario where it would be, it's incorrect to condition on $\op{W},$ because it would obscure the total causal effect of $\op{UTRS}$ on $\op{I}$ - precisely that portion mediated through $\op{W}.$
So the answer to your first question, as to what happens when you try something to be a confounder if it is already present, depends on the directions of the arrows. What causes what? In the first diagram, you should condition for $\op{A}$ because it is a confounder (assuming the model is correct, which it might not be). In the second diagram, you should NOT condition for $\op{W},$ because it is not a confounder but a mediator (assuming the model is correct, which it might not be).
The answer to your second question, as to whether general strength is a confounder, also depends on the arrow directions, and whether, if you want to include general strength in your model, it sets up a backdoor path or not. That's always the question: can you shut down all the backdoor paths? If so, and the variables you need are all measured, then you can identify the causal effect of $\op{UTRS}$ on $\op{I}.$ Otherwise not.
My answer here, you must understand, is more about pointing you to a framework in which you can answer your own questions, more than answering them myself. There are clarifications necessary in your problem before you can give a final answer.


