0
$\begingroup$

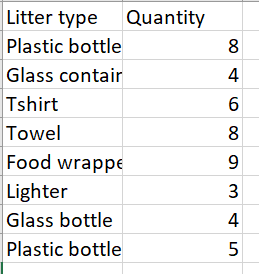
I have count data of litter found across a beach. In one column there is the litter type (plastic bottles, glass bottles etc.) and in the other the quantities found, such as below:

enter image description here

You can see that sometimes the same litter type comes back several times (bottles - I can sum the quantities of bottles and make it one data entry if required). In some cases I have extreme counts reported (e.g. 50 towels). Usually, the quantities range from 3 to 10. The aim here is to observe any significant difference between groups. Not knowing if I should go for a Poisson or negative binomial, I was looking to see if the mean of my data was equal to the variance:

enter image description here

Seeing they are not equal, and that sometime I have extreme counts, should I go for a negative binomial regression? In addition, I've run a Poisson regression on the data, and the omnibus test resulted in a p-value <0,05. Is that wrong in the case of a negative binomial regression needed? Many thanks

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Thanks for including a sample of your data. Honestly, it's not clear to me that a poisson or negbin model is appropriate for the sum of Litter Types, since this sum is across such disparate items. What additional information do you have about this information, e.g., why are there multiple entries for plastic bottles? Are multiple beaches or days of the week being aggregated? To me, these possible sources of variance need to be accounted for by any model. $\endgroup$
    – user234562
    Commented Jul 17, 2020 at 18:32
  • $\begingroup$ Thanks for your input. There are multiple entries because each entry was inputted by one single person. As there were 150 participants, I have 150 entries with different litter types and corresponding quantities. The idea is to observe any differences in quantities amongst litter types. As for now there is no more info being aggregated. $\endgroup$
    – Tim56
    Commented Jul 18, 2020 at 14:03

1 Answer 1

Reset to default
1
$\begingroup$

The Case Studies for the GENLIN procedure include a discussion of Poisson vs. negative binomial models and how to test to see which to use. See here.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.