Is there a reference that has the value of the following integral: $$F(a) =\int_{-\infty}^{a}xt_{\nu}(x)dx = \int_{-\infty}^{a}\frac{x}{s\sqrt{\nu\pi}}\frac{\Gamma\left(\frac{\nu+1}{2}\right)}{\Gamma\left(\frac{\nu}{2}\right)}\left(1+\frac{1}{\nu}\left(\frac{x-m}{s}\right)^2\right)^{-\frac{\nu+1}{2}} d x$$ where $t_{\nu}(x)$ is the pdf for Student's t distribution.
-
1$\begingroup$ I think your $F(a)$ is the mean of the truncated t-distribution with lower limited = $-\infty$ and upper limited = $a$. en.wikipedia.org/wiki/Truncated_distribution Except that yours isn't normalized. $\endgroup$– const-aeCommented Aug 28, 2020 at 15:27
-
1$\begingroup$ This is straightforward to evaluate in terms of the Student $t$ CDF and elementary functions, so why do you need a reference? $\endgroup$– whuber ♦Commented Aug 28, 2020 at 16:05
-
$\begingroup$ Yes, perhaps it is straightforward, but it's not a one-line long derivation. $\endgroup$– AlexCommented Aug 28, 2020 at 16:40
1 Answer
You're right: this is not (quite) a one-liner. But I can do a thorough job in just two lines by building on what you already know.
Let's make some standard preliminary observations to simplify the work and establish the notation.
Your $t_\nu$ is a location-scale family based on the Student $t$ distribution with $\nu$ degrees of freedom with a location parameter $m$ (which I will write as $\mu$ to make it clear it's a parameter and not a variable) and scale parameter $s$ (which I will write as $\sigma$). That is tantamount to a change of units of measurement of the original Student $t$ variable $Z$ to a new variable $X=\sigma Z + \mu.$ Thus, your integral becomes a linear combination of integrals with integrands $zt_\nu(z)$ and $t_\nu(z)$ (where from now on I will use "$t_\nu$" in the narrower sense of the standard Student $t$ density). The latter integrates, by definition, to the (standard) student $t$ CDF, which I will write $$T_\nu(z) = \int^z t_\nu(x)\,\mathrm{d}x.$$
This leaves us with the (genuine) problem of integrating $xt_\nu(x)$ for the standard $t$ density $t_\nu.$ The answer is contained in one of your previous questions, where you note
$$\frac{d}{dx}t_{\nu}=-\frac{\nu+1}{\nu+x^2}\,xt_{\nu}(x).$$
This can be rewritten (using the product rule of differentiation) in the form
$$\begin{aligned}\frac{d}{dx} \left(\frac{x^2+\nu}{1-\nu}\,t_\nu(x)\right) &= \frac{2x}{1-\nu}\,t_\nu(x) + \frac{x^2+\nu}{1-\nu}\frac{d}{dx}t_\nu(x) = \left(\frac{2}{1-\nu} - \frac{1+\nu}{1-\nu}\right) x t_\nu(x) \\ &= xt_\nu(x). \end{aligned}$$
In other words, you already know an antiderivative of $xt_\nu(x).$ The Fundamental Theorem of Calculus states this is the indefinite integral. All we need to compute is the constant of integration. But, provided $\nu \gt 1,$ the integrand $xt_\nu(x)$ decreases sufficiently rapidly as $a\to -\infty$ that the limiting value of its integral must be $0.$ Thus, the constant of integration is $0.$
Putting these results together gives for $\nu \gt 1$
$$\begin{aligned} \frac{1}{\sigma}\int^a x t_\nu\left(\frac{x-\mu}{\sigma}\right)\mathrm{d}x &= \sigma \int^{(a-\mu)/\sigma} z t_\nu(z)\,\mathrm{d}z + \mu \int^{(a-\mu)/\sigma} t_\nu(z)\,\mathrm{d}z \\ &= \sigma \left(\frac{\left(\frac{a-\mu}{\sigma}\right)^2+\nu}{1-\nu}\right) t_\nu\left(\frac{a-\mu}{\sigma}\right) + \mu T_\nu\left(\frac{a-\mu}{\sigma}\right). \end{aligned}$$
When $\nu \le 1,$ you may verify the integral does not converge.
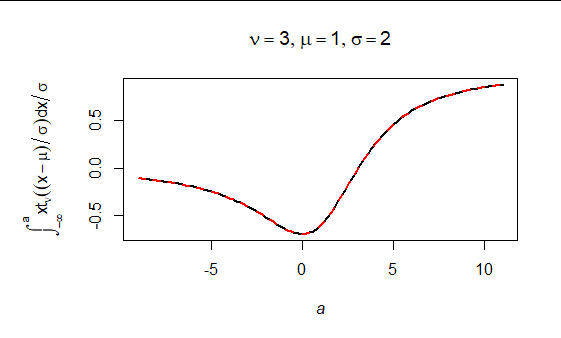
As a quick check, I computed this integral (as a function of $a$) both numerically and using the final formula. Here is a plot of the two computations, one in black and the other in red. They agree.