0
$\begingroup$

What are the Karush–Kuhn–Tucker conditions for $\min_x \frac{1}{2} ||x-u||_2^2:$ subject to $||x||_1\le c$

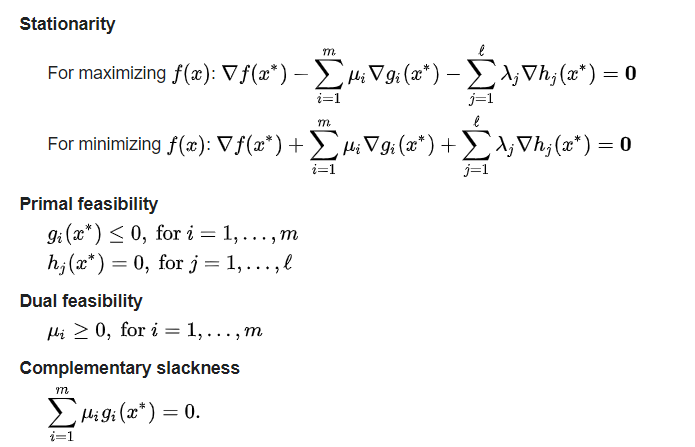
Apparently these are the conditions enter image description here

but it's not all that clear how this can be applied to above function, especially the stationarity portion.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Be sure to specify whether this is a homework problem for a class. You might get more answers on math.stackexchange as this is only tangentially related to stats. $\endgroup$
    – WetlabStudent
    Commented Nov 29, 2020 at 20:34
  • $\begingroup$ @WetlabStudent yes it's for a class. I don't normally post these kind of questions on Math stack exchange cause whenever I post something stats related it tends to get avoided and never receives answers for whatever reason, but when posted on here someone seems to be familiar with the concepts and provides an answer. $\endgroup$
    – user8714896
    Commented Nov 29, 2020 at 22:42
  • $\begingroup$ I've marked it self-study so folks know to give "helpful hints" rather than complete answers. $\endgroup$
    – WetlabStudent
    Commented Nov 29, 2020 at 23:37
  • $\begingroup$ I would translate one norm into two constraints $-c<x<c$ so $x<c$ and $-x<c$ then $g_1 = x-c<0$ and $g_2 = -x-c$ both differentiable functions. Then consider complentary slack saying either $\mu_i$ is 0 or constraint $i$ is binding. Consider then possibilities - if $\mu_i>0$ then constraint is binding: Can both constraints be binding? When will one constraint be binding? When will none be binding? $\endgroup$
    – Jesper for President
    Commented Nov 30, 2020 at 3:06

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.