3
$\begingroup$

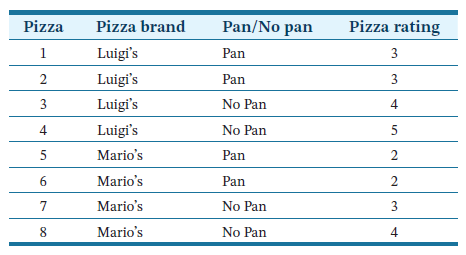
An ANOVA analysis is done on the quality of pizza in two settings:

  1. pan vs. no pan while blocking on pizza brands,

  2. one brand vs. the other while blocking on using a pan or not.

enter image description here

Will the F-statistics change for Pan and Brand across the two settings?

I just don't understand how the blocking would work in this scenario. Can someone demonstrate how you would group pizza ratings in both settings?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

What you have is a $2^2$ factorial design twice replicated. That means that the two factors, that you call blocks, are orthogonal. So if you compare brands by means of ratings, the comparison is balanced with respect to the other factor. Likewise switching roles. You could fit a linear regression, but we can get the same results by simple arithmetic, thanks to ortogonality.

Present mean ratings as a $2\times 2$ table $$ \begin{array}{rrr} \hline & Pan & noPan \\ \hline Luigi's & 3.00 & 4.50 \\ Mario's & 2.00 & 3.50 \\ \hline \end{array} $$ Now you can do the comparisons by mental arithmetic. The row-wise and col-wise differences are almost the same, indicating no interaction.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.