Is my understanding correct. If P1-P3 are unobserved, then I can not use Z as an instrumental variable. However, if they are available and I can adjust for P1-P3, then Z becomes a valid instrumental variable?
The output of dagitty.net
Instruments and conditional instruments:
Z | P3, P2, P1 (this means that Z is a valid instrument if I control for P1-P3?)
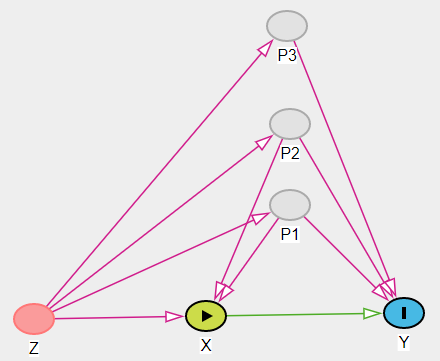
To verify for yourself that that what you are saying is correct, it suffices to precisely state the definition a (conditional) instrument and then use the graph to convince yourself that controlling for $P_1, P_2, P_3$ would suffice to make $Z$ a conditional instrument.
More precisely, we say that $Z$ is a conditional instrument for $X$ given some set of covariates $W$ if it satisfies the following two conditions
$$Z \perp Y | W, do(X) \quad \text{and} \quad Z \not\perp X | W$$
The first condition is the "exogeneity/exclusion" condition and roughly means that $Z$ is independent of any common causes of $Y$ once $W$ is controlled for, and it only affects $Y$ through its effect on $X$. The second condition is the "relevance" condition that $Z$ has some effect on $X$. Given these definitions of what an instrument is, you should be able to verify both conditions above by appealing to d-separation when $W$ is taken to be the variables $P_1, P_2, P_3$.
I should add though that if actually believe that the above graph completely captures the causal structure among $Z, P_1, P_2, P_3, X, Y$, there is little reason to actually use $Z$ as an instrument for $X$. Specifically, you use an instrument when you have an $X$ which is potentially endogenous in the sense that you are worried that there are some variables, called confounders (in this case, the $P$'s), that have causal effects on both $X$ and $Y$ that you cannot observe and therefore cannot control for, but you have an instrument $Z$ that affects $Y$ only through its effect on $X$ and does not have itself confounders with respect to $Y$. But in the above example, if you controlled for $P_1, P_2, P_3$, you would already be controlling for all confounders and therefore already have enough to estimate the causal effect of $X$ on $Y$ directly (more formally, one could check that $P_1, P_2, P_3$ together satisfy the backdoor criterion relative to $X, Y$), so no instrument is necessary. Moreover, instruments are imperfect in the sense that they typically only identify local or weighted causal effects, so not only is using $Z$ as an instrument unnecessary in this setting, but doing so would actually be strictly worse than estimating the causal effect directly.
