What statistics can be used to analyze and understand measured outcomes of choices in binary trees?

Question

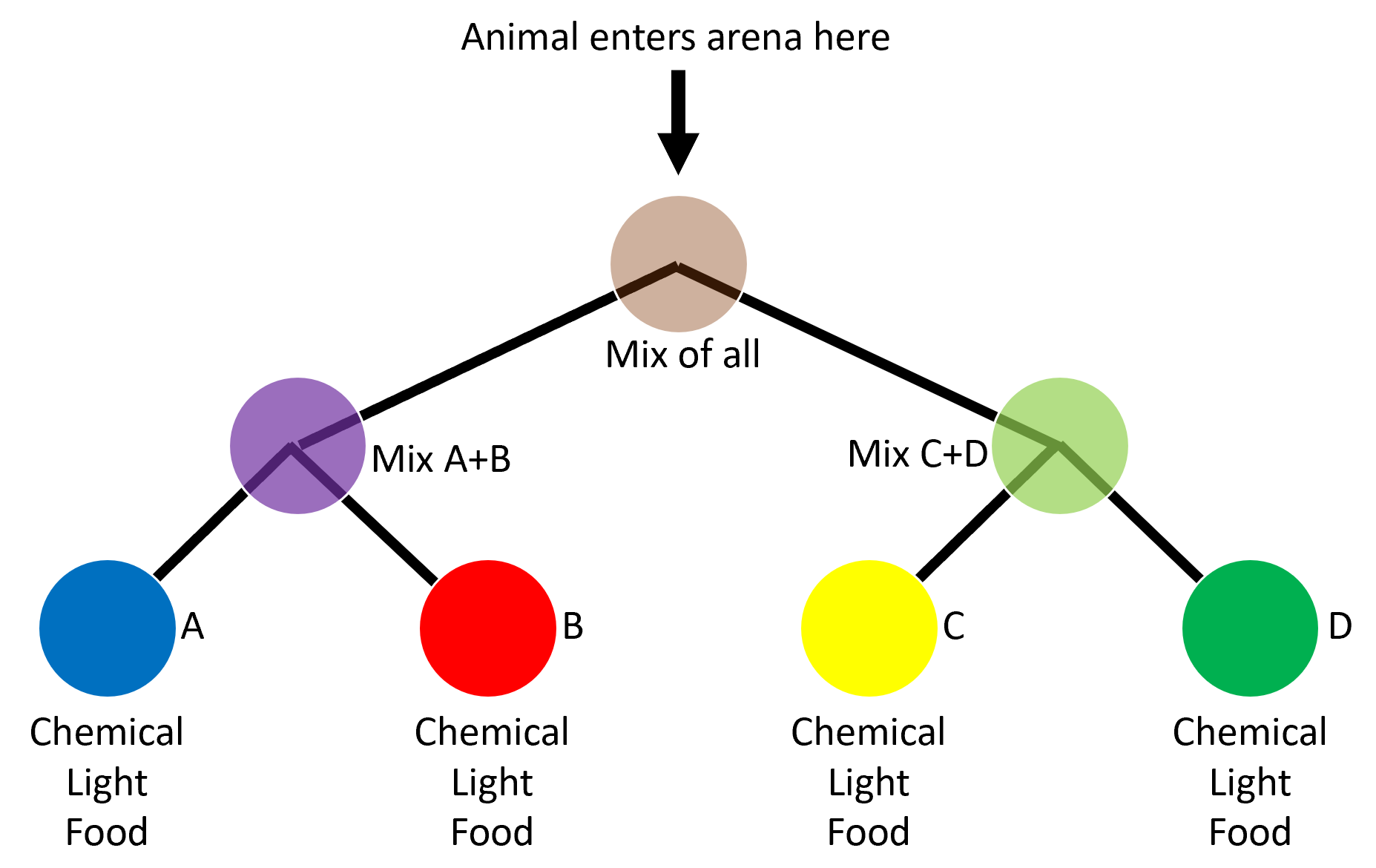
I am conducting biological research on animal behavior. There is an arena set up like a binary tree. End nodes are sources of food (e.g. smells) or stimuli that can mix. The animal (or a group of animals) enters an arena from the top, and navigates and makes choices based on the stimuli that reach it, always making a binary choice at each node, until it reaches the lowest node. The animal at first finds itself in a complex mixture, and at each fork makes a choice between simpler mixtures. A visual aide:

The arena size (binary tree height) is not specified yet, it will be around 2, 3 or 4. The idea is to understand or disambiguate the contribution of each of the smells by which choice an animal makes, at every fork of the tree, and where it ends up eventually. The end nodes will be randomized repeatedly and information will be collected about the choices an individual or group of animals make within this arena multiple times.

Question: what kind of statistics or math or tools can be used to understand the 'weight' or contribution of each end node to the observed animal choices? It seems to me the design of the experiment allows for several mathematical/statistical approaches. Unfortunately I do not know where to begin or what to even describe this problem as to learn more about it.

Answer 1

Thanks to Jake Westfall for alerting me of this question. It does indeed sound like something that could be modelled with a multinomial processing tree (MPT) models.

The experiment in your figure would provide a you with a multinomial distribution with four categories ($C_A, C_B, C_C, C_D)$, which provides three independent data points (i.e., maximum parameters). A simple saturated MPT model for the categories could be:

$ Pr(C_{A}) = m_{A,B}*t_A\\ Pr(C_{B}) = m_{A,B}*(1 - t_A)\\ Pr(C_{C}) = (1- m_{A,B})*t_C\\ Pr(C_{D}) = (1- m_{A,B})*(1 - t_C) $

As in any MPT model, the parameter represent (conditional) probabilities that a certain event occurs. Here the three parameters are:

• $m_{A,B}:$ do animals choose the A & B mix over the C & D mix?
• $t_A:$ do animals choose the A over B?
• $t_C:$ do animals choose the C over D?

In a typical MPT task, you would have other trees that share the same parameters. That allows you to fit a non-saturated model which provides some information whether the overall model and its assumption fits the data.

However, in the present example there are four different and apparently unique stimuli. So sharing parameters across trees might not be easily be possible. One possibility might be to consider a baseline stimuli. For example, consider that stimuli B and D are the same. Then you could consider other experiments/trees in which A & B also occurs in one tree, but you have a different comparison in the other branch, say E and B. This tree would now share the same $t_A$ parameter as the tree you have, but all other parameters are different. This would allow you to estimate the various probabilities while allowing to test if the assumption that say $t_A$ is the same across tasks holds.

Also, once you have specified the model equations as shown in the example, their exists easy to use software tools to fit such models, TreeBUGS, which even allows for a hierarchical Bayesian approach allowing for one random-effects term such as animal. They also have a good introductory paper: https://cran.r-project.org/web/packages/TreeBUGS/vignettes/Heck_2018_BRM.pdf