This paywalled article shows that the difference of two i.i.d. random variables is unimodal and symmetric if the distribution of the random variables is unimodal. Is there a non-unimodal distribution such that the difference isn't unimodal?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ Non unimodal difference when RV is non unimodal itself is pretty easy. May you be asking for unimodal difference when RV is non unimodal instead? $\endgroup$– gunesCommented Apr 20, 2021 at 7:26
-
3$\begingroup$ @gunes No the first thing you mentioned (the 'easy' one) is what I was after. But when you described it as easy, I realized it was (eg bimodal betas distributions do the trick). $\endgroup$– Hasse1987Commented Apr 20, 2021 at 14:01
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
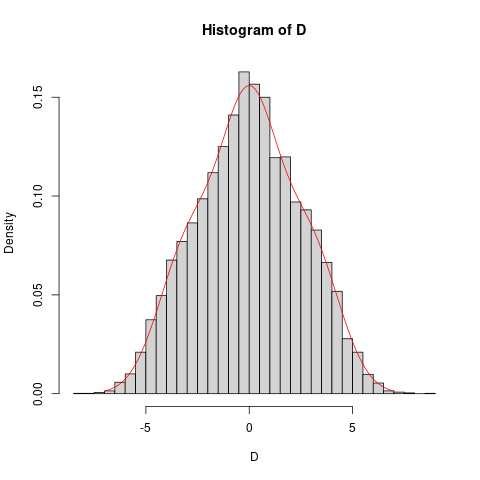
Example of non-unimodal distribution where the distribution of difference is unimodal:
Let $X$ and $Y$ be observations of a normal mixture distribution with expectations 0 and 3, standard deviations 1, and mixture proportions 0.5, 0.5. Below is a histogram of the difference distribution based on 10000 samples. It surprised me that it is unimodal
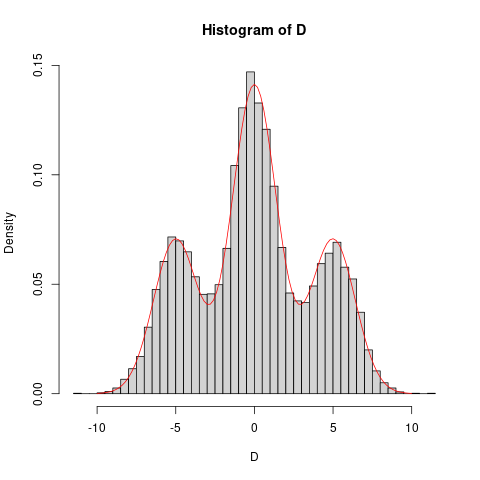
Example of non-unimodal distribution where the difference distribution is not unimodal:
As above, the only difference is expectations 0 and 5:
answered May 15, 2021 at 23:50