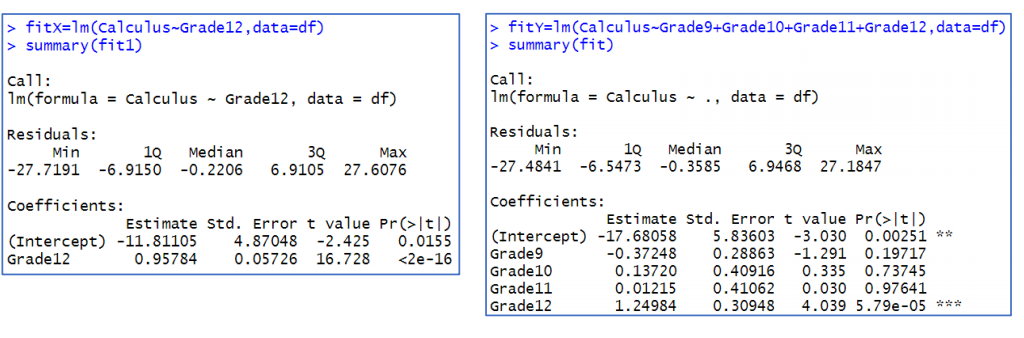
Context of the question:
Two professors want to look at the effect of students’ high school math marks on their marks in their first calculus course. All marks are between 0 and 100. Using data from 1000 students, Professor 1 fits a simple linear regression model with calculus mark as the response and mark in Grade 12 math as the predictor. Professor 2 fits the same model but also includes marks in Grades 9, 10, and 11 math as predictors. Some output associated with the fitted models is given below. What is the most likely reason that the standard error of the estimated effect of Grade 12 mark is so much higher in Professor 2’s output than in Professor 1’s output?
I don't understand why the addition of grades 9-11 so heavily impacts the standard error of the estimated effect.