Fixed effects in a cross-sectional data

Question

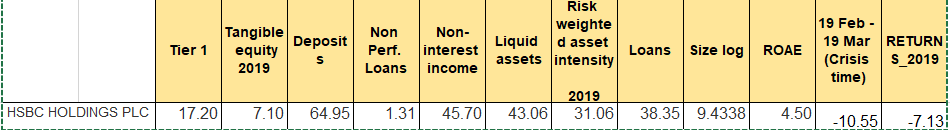
I'm working with research that has cross-sectional data. I have collected information about publicly-listed banks in many countries. For example, for each bank I collected the following information:

I have 619 banks in 58 different countries. In my research, I want to test how variations in bank characteristics (Tier 1, Tangible Equity, etc.) affected bank stock returns during crisis time. My equation is as follows:

BPb,c = 𝑎 + 𝛽1RETURNS_2019b + 𝛽2TIER_1b + 𝛽3DEPb,+ 𝛽4NPLb + 𝛽5NONIIb + 𝛽6LIQASSb + 𝛽8SIZEb + 𝛽9DENb + 𝛽10ROAEb + 𝛽11 LOANSb + 𝛽3*TANEQb + 𝛾c + ub,c

Where BPb,c is the performance of a bank b in country c. The coefficients 𝑎, 𝛽, represent vectors of coefficient estimates and ub.c is the error term. 𝛾c - country fixed effects.

In all the literature I have read, fixed effects are applied to panel data models. However, following Beltrati and Stulz (2012), which to my understanding, has cross-sectional data as well, they apply fixed effects and use standard errors clustered by country.

Is this approach using country-fixed effects and clustering error by country (with cross-sectional data) logical? Also, perhaps someone could advise how to implement this model in Stata.

Answer 1

I have not looked at the paper, but in general, you can only apply fixed effects to panel data sets. The reason the it's called "fixed effects", is that you assume certain effects to be fixed or constant over time.

E.g. in your case, you would have to have several observations for each bank and could then avoid omitted variable bias due to unobserved but time-invariant, country-specific effects.