Positive expected value for lottery

Question

As far as I know, to decide either you should enter a bet or not, you should get the expected value of that bet

I was wondering if the lottery has a very high expected value, is it wise to join?

There is a real life lottery with this inputs

Choose 5 numbers out of 50

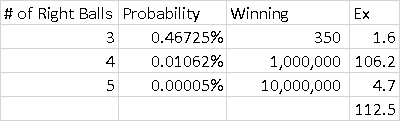
If you get 3 right you win 350

If you get 4 right you win 1000,000

If you get the 5 numbers right you win 10,000,000

Entry fee is 35

So Expected value is

so total of 112.5- 35(entry fees) = 77.5

This is a very high expected value, i wonder if i did the math wrong?

it was like simple probability

3 winning balls should be
(combin(5,3) * combin(45,2)) /combin (50,5)

And so on did I calculate it wrong? or it's just too good ? and if the expected value is more than double the entry fees, is wise mathematically to join for a long run?

Noting that for i know that for even wining 4 numbers, probability to lose is 99.989%

on the long run for 52 times for example, the probability to lose them all is 99.989%^52 = 99.449%

I'm just confused how the expected value is too high, yet by intuition it seems the right decision is not to join even for a long run Or how should I think about it?

Edit : I guess the point is the 1 Million prize will be shared between winners, but i don't know how should i calculate the expected value in that case?

Answer 1

The expected value calculation when the pot gets to ~$20$ million or more has to take into account the returns from investing the winnings. If you win a pot of $20$ million and get about $10$ million after tax (being ver conservative about the tax rate here), you could reasonably expect to earn $4\%$ per year, which is $400$k per year. If you are financially responsible, a win $20$ million is enough for you and your kids to be financially independent.

Answer 2

I simplify the lottery a little bit. Let's say the players can win a fix prize $W$, no matter how many players participate in the lottery. We do not regard any fee. Each player can win this prize with a probability of $p$. If n players win, then the prize is equally distributed among the players. W.l.o.g. we calculate the expected value for 2 players. They win in total $W$ if at least one of the players win. Thus the expected value is

$$E^{(2)}(W)=W\cdot \left( p^2+2\cdot p\cdot (1-p)\right)=W\cdot (1-(1-p)^2)$$

We can generalize the result

$$E^{(n)}(W)=W\cdot (1-(1-p)^n)$$

Now the fix prize W has to be equally distributed amoung the $n$ players. Thus the expected value for one player is $E^{}(W)=\frac{W}n\cdot (1-(1-p)^n)$. We can look at some interesting values.

• $\lim\limits_{n\to \infty} \frac{W}n\cdot (1-(1-p)^n)=0$. If (theoretically) the number of players goes to infinity the expected value is $0$.

• If only one player participate at the lottery then the expected value is $W\cdot (1-(1-p)^1)=p\cdot W$.

If the prize W is increasing in some way by the number of players $n$, then this can be taken into account.