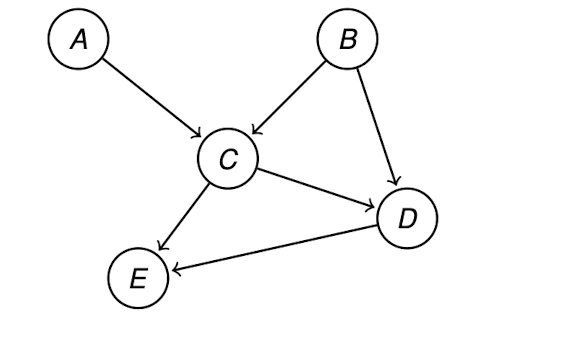
In the DAG above, we have $A$ conditionally independent of $E$ when $C$ and $B$ are observed (that is $A\perp E|B,C$), but not when only $C$ is observed (that is $A\not\perp E|C$). I don't have a good intuition on why this is true, it feels to me like it should still be conditionally independent.
Could anyone give me a good explanation of why my intuition is wrong?
Flow of dependencies in DAGs are determined by the d-separation criteria, and two variables are d-separated if and only if every path (without considering the orientation of the arrows) is blocked.
Now in your specific example, by conditioning on $C$ you block the path $A \rightarrow C \rightarrow E$ but you also open the path $A \rightarrow C \leftarrow B \rightarrow D \rightarrow E$, therefore not all paths are blocked and we got $A\not\perp E|C$.
On the contrary, by conditioning on both $C,B$ or $C,D$, all the paths between $A$ and $E$ are d-separated.
