1
$\begingroup$

I have 2 (monthly) time-series that look like this:

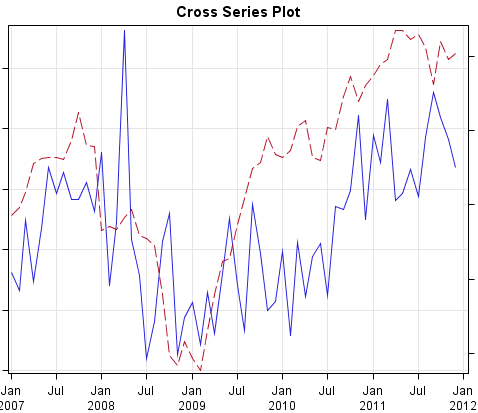
cross plot of 2 series

Economical intuition suggests that they are positively related and I can see this on the plot but if I compute correlation between their log-returns $\ln x_t/x_{t-1}$ and $\ln y_t/y_{t-1}$ this correlation is -0.04 this is basically zero and not statistically significant for my data size (~60 points).

How can it be?

One may say that series are cointegrated $y_t = a x_t +\varepsilon_t$, but then returns should follow $\Delta y_t = a \Delta x_t +\Delta \varepsilon_t$ and correlation between returns would also be significant. So if I see zero correlation between returns, there is no cointegration between levels as well - right?

So does this zero correlation means that there is no relation between series? If yes - why do they follow each other so closely.. If no - how to quantify this relation if correlation between diff'ed series is ~0 and cointegration tests for original series are inconclusive.

EDITS:

-- added cointegration -> correlation link to address @AlecosPapadopoulos question.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ Why don't you run formal co-integration tests instead of "saying" that the series are co-integrated? $\endgroup$
    – Alecos Papadopoulos
    Commented Oct 25, 2013 at 8:40
  • $\begingroup$ @MichaelMayer Thanks for suggestion! Not quite clear what do you mean by "strong time interaction" - could you explain a bit more? $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 4:11
  • $\begingroup$ @AlecosPapadopoulos If series would be cointegrated - their returns would also be correlated - right? If $y_t = a x_t +\varepsilon_t$ with $\varepsilon_t$ stationary i.e. series are cointegrated then same relation holds for diffed series $\Delta y_t = a \Delta x_t + \Delta \varepsilon_t$ - right? So if I see zero correlation between monthly returns then cointegration should not also appear? $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 4:17
  • $\begingroup$ @AlecosPapadopoulos I tested for cointegration with 2 tests - both give inconclusive results. Engle-Granger test in SAS AUTOREG cant reject that there is a unit root in residuals (so no cointegration). Stock-Watson test for common trends in SAS VARMAX can't reject that there are common trends $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 7:38
  • $\begingroup$ I should have said "strong confounding effect of time", thus I removed my comment. What I had in mind is very closely related to the following post: stats.stackexchange.com/questions/71005/… $\endgroup$
    – Michael M
    Commented Oct 28, 2013 at 18:23

2 Answers 2

Reset to default
1
$\begingroup$

Your measure is a short-time-scale measurement; note that you are only looking at the (log) differences between successive time stamps. There is enough short-time-scale noise that is is masking the longer-term, $O(1 year)$, timescale correlations in the data.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks a lot, this indeed gives some insight. How would you suggest to measure longer-term correlations? I can't aggregate data by years and compute correlation between yearly values - there are only 5 years. I tried to consider rolling 3,6,12-month differences: $\ln(x_t)-\ln(x_{t-3})$ and $\ln(y_t)-\ln(y_{t-3})$ etc. Correlation between 3-m diff: 0.12, 6-m diff: 0.32, 12-m diff: 0.49! Seems to work, but those diff'ed series become less and less stationary... and then correlation becomes not a valid measure? Ultimately I could compute corr for original series which will be positive. $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 4:07
  • $\begingroup$ So I guess, there is some "optimal value" of "smoothing" window when correlation is not affected by high frequency noise but diffed series are still close to stationary. Do you know any proper way to determine this? Or, maybe there is another way to quantify the relation in above series? $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 4:10
1
$\begingroup$

This is a textbook example of spurious time series regression. The levels are highly correlated, but the differences are not. This happens when we have two independent random walk processes. To make sure that this is really the case, check that the residuals from the level regression have unit-root and that the residuals from the difference regression do not have it.

Improve this answer
$\endgroup$
5
  • $\begingroup$ ADF test can't reject possibility of unit root in residuals for levels, so - no conclusion. I'm pretty sure residuals from diff'ed regression will not have unit root. But I don't think this is a spurious relation - in my case it has some economic meaning and visually series look following each other closely. I'd rather believe in @Dave's suggestion above that correlations at different time-scales can be different and high-frequency noise affects this particular realization of sample correlation between diff'ed series. $\endgroup$
    – Kochede
    Commented Oct 28, 2013 at 8:56
  • $\begingroup$ Did you test for cointegration? Do the residuals of level regression have unit root? If you say that there is an economic meaning of the relationship, maybe there are some articles which investigate it. This might give you an idea of what kind of model is apropriate. $\endgroup$
    – mpiktas
    Commented Oct 28, 2013 at 11:57
  • $\begingroup$ Somehow I assumed that your time series are unit roots, what do the ADF test say about levels? $\endgroup$
    – mpiktas
    Commented Oct 28, 2013 at 11:58
  • $\begingroup$ I tested unit-root of residuals and levels: in all cases ADF-test can't reject that there may be unit-root. So correlation is 0, cointegration also seems oof-table, then it still puzzles me why those series look following each other (although at different scale) $\endgroup$
    – Kochede
    Commented Oct 30, 2013 at 10:16
  • $\begingroup$ I'm concluding that this relation is spurios, but what @Dace said above about different correlations at different time-scales and high-frequency noise masking lower-frequency correlation may be the key. $\endgroup$
    – Kochede
    Commented Oct 30, 2013 at 10:18

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.