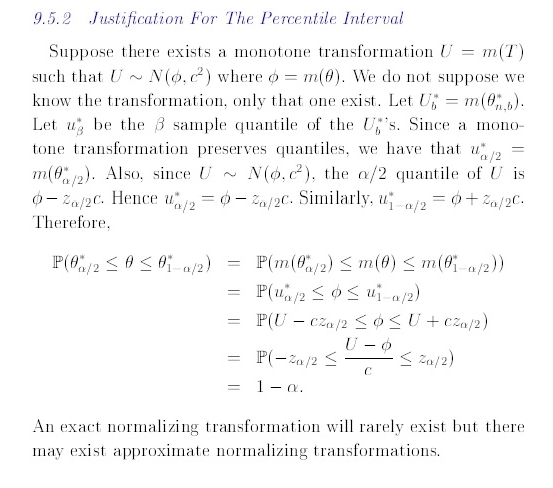
The following is a proof of the validity of the (bootstrap) percentile interval taken from Larry Wasserman's "All of Statistics". $\theta^*_{\alpha/2}$ and $\theta^*_{1-\alpha/2}$ denote the respective quantiles of the bootstrap of our estimator $\hat {\theta}$.
Could you please take a look at the proof below and explain to me where the third line of the derivation comes from? How does $U$ enter the derivation? Is there perhaps a mistake there?
Thank you in advance.
I think the source of confusion is the in the two sentences before the derivation. Before the "Hence", I would add: "Suppose also that conditional on $U$, $U^*_b \sim N(U, c^2)$." Then, the next sentences should be, "Hence, $u_{\alpha/2}^* = U - z_{\alpha/2} c$ and $u_{1-\alpha/2}^* = U + z_{\alpha/2} c$."
A transformation $m$ that satisfies both the original "Suppose" and my addition will rarely exist. However, it will usually exist asymptotically as the sample size grows. This is what Wasserman is alluding to in his last sentence. Essentially, $m$ existing means that the true distribution of your estimator, $\hat{\theta}$, and the bootstrap distribution of $\theta^*_{n,b}$ are the same.
