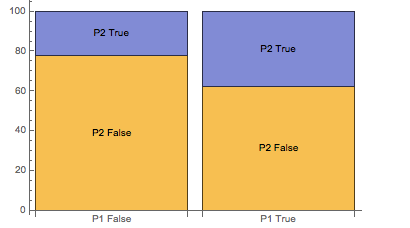
- Is there any correlation? Using 2x2 contingency table analysis, the Phi coefficient of association is 0.14, with chi-squared = 74.08 and n = 4040. So, yes, there is some correlation (or association).
- Is there any causation? I don't think it will be easy to determine that philosophically, statistically, or otherwise. There is simply not enough information to know how these numbers came to be. I think you need to see if this data is observational or experimental; some people seem to believe and/or argue that you cannot make causal claims from using observational data. I think you need to pick a causal framework (one that defines what is causality) and see if the causal calculus (if any) available in that framework will allow you to draw a causal link between these two variables.
Note these sayings
- Correlation does not imply causation.
- Causation leads to correlation.
So, it seems to me, a causal relationship should manifest (or cause) a correlation relationship. But, just because you see a correlation relationship, does not mean there is a causal relationship. Kind of like (as an intuitive example), fire leads to smoke, but observing smoke may not mean there is fire.
So, I believe, you may have a causal relationship, it is not ruled out yet, because you have a correlation relationship. But it is not definitive, whether you have or do not have a causal relationship. Remember, stock prices have been shown to be correlated with moon cycles, yet, is there a causal relationship?