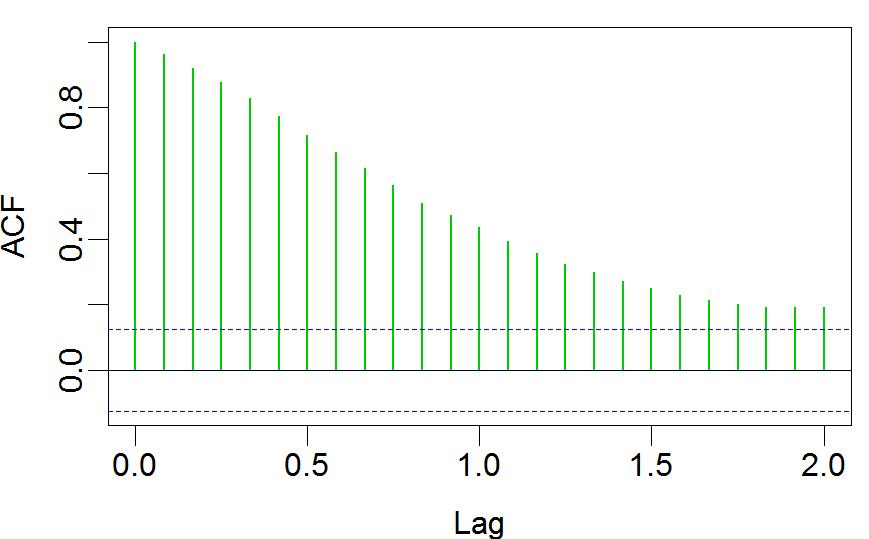
I was thinking, from the ACF it looks like a nonstationary process. Or is it an AR process? From the PACF, does that mean it is AR(1)? There are 2 significant spikes in the PACF. I'm confused.
$\begingroup$
$\endgroup$
2
-
1$\begingroup$ what sort of data is it on? $\endgroup$– Jan SilaCommented Oct 18, 2016 at 8:39
-
$\begingroup$ it is a time series data of a commodity price (natural log of real prices not nominal). Its sugar $\endgroup$– user7035336Commented Oct 18, 2016 at 9:20
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
Firstly, you should have integer values on the x-axis...
The ACF suggests there is long memory present in the data set. You might try to estimate it - have a look at the options.
In terms of PACF, it suggests what is the order of autocorrelation in the series, but in this case the spike is at 10th and personally, I would assume that AR(1) with $x_{t-10}$ won't really explain much. But it depends what sort of data is it on, maybe it makes sense...
-
1$\begingroup$ Generally noninteger values on the $x$-axis are not a problem. This could be monthly data with a period of 12, then a
1on the axis stands for 1 year. Also, why long memory? Looks like ACF has an exponential (rather than linear) decay, hence short memory. $\endgroup$ Commented Oct 18, 2016 at 8:52 -
$\begingroup$ Is there evidence of the time series being Non-stationary, judging from the acf? $\endgroup$ Commented Oct 18, 2016 at 9:07
-
1$\begingroup$ @user7035336 Yes, actually, there could be a unit root (and then long memory, indeed). $\endgroup$ Commented Oct 18, 2016 at 9:24
-
$\begingroup$ @user7035336 if you are doing it on log prices, then I bet you made a mistake - I was gonna say you modelled the ACF on prices as they are always non-stationary
~unit root and similar shape :) You want to take natural logarithm and then difference those to get logarithmic differences - thats' what finance works with. Try that and have a look at the ACF and PACF again. $\endgroup$– Jan SilaCommented Oct 18, 2016 at 11:24