1
$\begingroup$

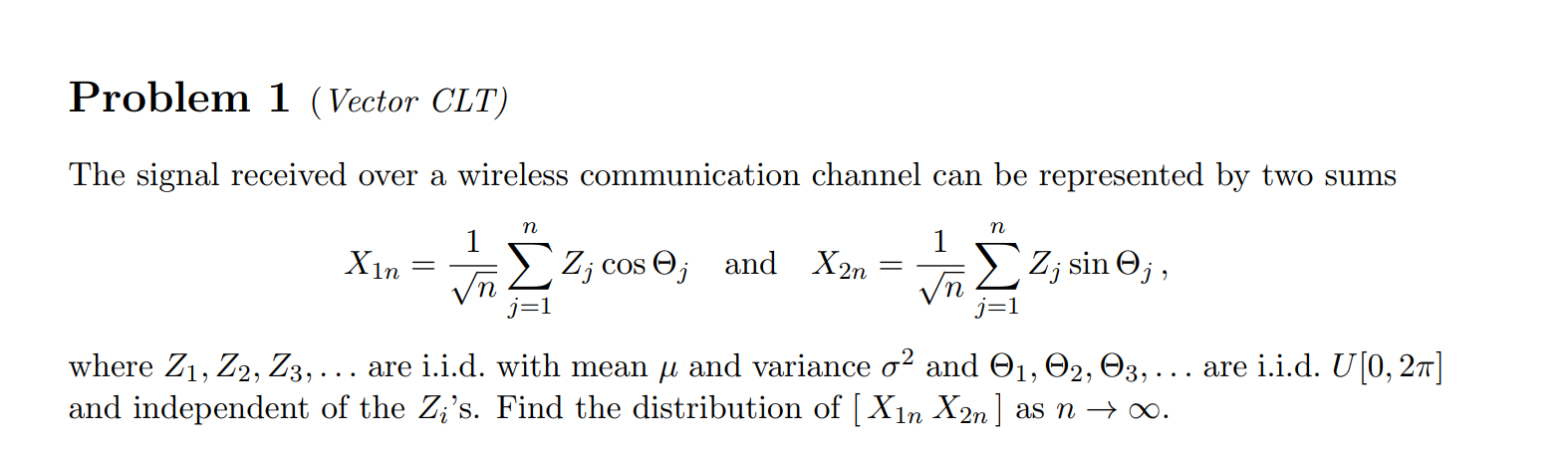
Given Problem 1) problem

To get the variance and covariance the following steps are taken: variance steps

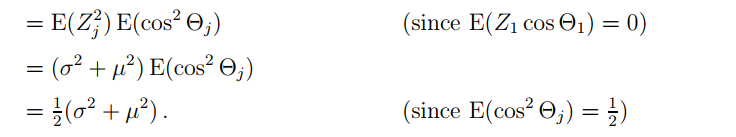
In the step below to calculate E[Z^2] how do we approach this without a known pdf? For completeness would finding E[cos(theta)^2] be the same set of steps? But this is different because at least we know that theta has a uniform distribution? confusion

Maybe what I'm looking for is a trick, theorem or a property? I'm not completely sure how to fill my misunderstanding in this single step. Otherwise everything else in the problem makes complete sense.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

$E[Z^2] = Var(Z) + E[Z]^2 = \sigma^2 + \mu^2$

The mean and variance of Z is given in the question.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.