4
$\begingroup$

I am a medical doctor working on a project within the field of pharmacokinetics. A lot of detailing is required, so please bear with me.

The aim is to describe the distribution of half-lives of a drug in a population, using data that was not intended for this specific purpose. I would like to know whether my chosen strategy is sound, and I also have some specific questions. I am not a mathematician, so please forgive me if my take on this is non-standard.

Blood samples were collected from hundereds of individuals. Two consecutive blood samples were taken 30 minutes apart from each individual. For simplicity, we name the drug concentrations in the first and second blood sample $C_1$ and $C_2$, respectively, and the time between blood samples $\Delta t$.

We are assuming that the drug is eliminated from blood according to first-order kinetics. As such, the relationship between $C_1$, $C_2$ and $\Delta t$ can be described by a simple exponential decay function:

$$C_2=C_1\cdot e^{-k\cdot \Delta t}$$

In each case, the rate constant $k$ is calculated as follows:

$$k=\frac{\log C_1-\log C_2}{\Delta t}$$

Half-life ($t_{1/2}$) can be calculated from $k$: $$t_{1/2}=\frac{\log 2}{k}$$

The main challenge is that $C_1$ and $C_2$ are subject to a random error, most importantly the imprecision of the chemical analysis used to determine drug concentrations. The coefficient of variation (CV) of the analysis is 9 %, such that each measured value of $C_1$ and $C_2$ can be assumed to be the true value $\pm$18 % (approximately).

In order to check whether there is a significant difference between $C_1$ and $C_2$, the critical difference $D$ was calculated using the method described by C. G. Fraser:

$$D = \sqrt{2}\cdot Z\cdot CV \approx 0.25$$

Z is the Z-score for a bidirectional difference to be significant with p = .05, approximately 1.96. Expressed in words, we assume that there is a true difference between $C_1$ and $C_2$ if their absolute difference is greater than 25 %. In more than 90 % of all cases, there was no significant difference. The mean difference was only 4.4 %.

The most obvious implication of this is that the values of $k$ and $t_{1/2}$ calculated from $C_1$ and $C_2$ in individual cases cannot be trusted to be anywhere near the true values. The distributions of calculated $k$ and $t_{1/2}$ are severely distorted or 'smeared out' compared to the 'true distributions' that we would have found if there were no random error.

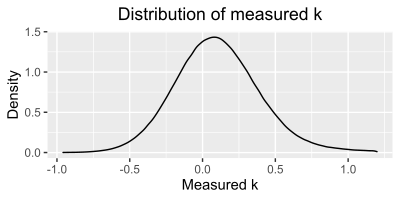
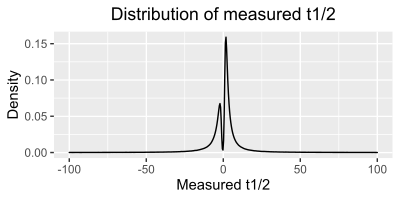
The distribution of calculated ("measured") values look like this (with the x-axes cropped to show the most relevant areas; there were many extreme outliers well beyond what is shown):

Distribution of calculated kDistribution of calculated t1/2

$\bar{X}_k \approx 0.120$ and $s_k \approx 0.360$.

$\bar{X}_{t_{1/2}}$ is in fact infinity and $s_{t_{1/2}}$ impossible to calculate due to the presence of infinite values.

We can make some, although very limited, assumptions about the a priori distributions of $k$ and $t_{1/2}$. They must have non-zero, positive values. They are very likely to have marked positive skewness, and especially $t_{1/2}$ is expected to have a substantial right tail. They may be lognormally distributed, but we cannot be sure of this. Based on previous litterature about the drug, $t_{1/2}$ can have values anywhere between 0 and 70, maybe even higher.

Given the analytical CV of 9 % and $\Delta t$ of 0.5 hours, we can simulate e.g. a million calculated values of $k$ if there were no true difference between $C_1$ and $C_2$ and all the observed difference was due to random error. Using R:

(log(rnorm(1000000, 1, .09)) - log(rnorm(1000000, 1, .09))) / .5

The SD of the simulated values, $s_{analytical}$, is 0.257.

Question 1: Assuming that biological variability and analytical imprecision are only two sources of variability of calculated $k$, is it justifiable to calculate the 'biological SD' from the 'total SD' (0.36) and the simulated 'analytical SD' (0.257)?

$$s_{biological} = \sqrt{s_{total}^2-s_{analytical}^2} \approx 0.252$$

Question 2: Is there an easy way to calculate $s_{analytical}$ directly, without using simulation?

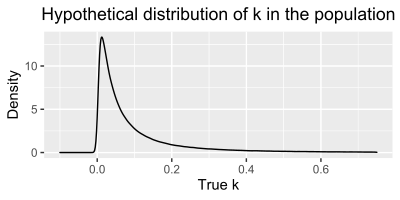
Question 3: Making the crude assumption that $\mu_k = \bar{X}_k = 0.120$ and $\sigma_k = s_{biological} = 0.252$, and that $k$ is lognormally distributed, we get the following distribution of 'true' values of $k$:

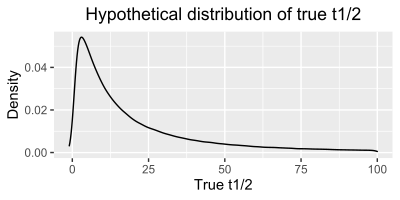
enter image description here

Converting this into $t_{1/2}$ ($\log 2 / k$), we get:

enter image description here

Now, if I simulate a large number of $k$ and $t_{1/2}$, I find that $\mu_{t_{1/2}}\approx 31$ hours. On the other hand, the $t_{1/2}$ corresponding to $\mu_k$ is $\log 2 / 0.12 \approx 5.8$ hours.

What is the mathematical explanation why $\mu_{t_{1/2}}$ is apparently unrelated to $\mu_k$ even though $t_{1/2}$ and $k$ are two sides of the same coin? Despite the fact that $\bar{X}_k$ seems to be a useful approximation of the central tendency of $k$, it is unclear to me what the $t_{1/2}$ calculated from it actually tell us.

Question 4: If all we have is $\bar{X}_k$ and $s_k$ and the only assumption we make about the true values of $k$ or $t_{1/2}$ is that they are positive numbers, can we know anything about the central tendency and spread of $t_{1/2}$ at all?

Improve this question
$\endgroup$
3
  • $\begingroup$ What you propose is close to a Bayesian approach: start by stating broad, physically realistic assumptions about what the parameter values might be, then use the data to refine your estimates. Statistical Rethinking is a superb introduction. In your case, the many negative "measured" $k$ values suggest that your sampling times aren't well chosen, as @AdamO suggests--perhaps they're not during an exponential-decay phase, or sampling times are too close together. $\endgroup$
    – EdM
    Commented Oct 16, 2021 at 14:32
  • $\begingroup$ Is the model assumption that $k$ is fixed, with observed variability due to sampling errors on $C_{1,2}$, or that $k$ itself varies in the population? I wasn't sure if the "Hypothetical distribution of k in the population" was exactly that latter thing, or more an a priori expectation. If $k$ varies from person to person, and there's only one noisy measurement for each person that doesn't peg $k$ with much precision, I worry the problem may be underdetermined? $\endgroup$
    – jwimberley
    Commented Oct 16, 2021 at 14:55
  • $\begingroup$ $k$ varies in the population, and that variation is precisely what I am trying to extract from the noise caused by measurment imprecision. The reason for the large number of negative $k$s is that, based on a priori knowledge of the drug, the true difference between $C_1$ and $C_2$, can be less than 1 % in some cases, which will of course "drown" in a measurment CV of 9 %. So the individual measurments of $k$ are very misleading. The "hypothetical" distributions are plausible but not necessarily correct distributions of true $k$ values that will give rise to results similar to what we have. $\endgroup$
    – Peder Holman
    Commented Oct 16, 2021 at 17:40

2 Answers 2

Reset to default
5
$\begingroup$

I can't follow this approach 100%, but it's a little surprising because the approach seems like nothing I've done in pharma. When we do pharmacokinetic analysis, we sample blood concentrations of drug at several timepoints, at least 5 times. These data are input into a statistical software package called winnonlin which is basically a fancy non-linear least squares that fits a standard dose concentration curve to the data. When data has to be pooled across many subjects or many administration time-points, we test for something called dose proportionality by fitting the predicted curves and adding random subject/visit level effects. If the test for the random effect is non-significant, we say that the doses are proportional.

The point is that the $k$ parameter seems to be too random. A dose concentration curve has to start at 0 mechanically, quickly ramp up, then taper off logistically. If the time point 1 is planned for the Cmax (the highest concentration) time point, it will be slightly under and slightly over if the dosing is non-proportional. I wonder if this is the issue. It might explain why such a large number of $k$ are close to 0, and why the half lives are non-sensiscal.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I agree that this approach seems very unusual. Some clarification is needed. The pharmacokinetics of the drug in question has already been well characterised in previous litterature. The blood samples used in this study were acquired by the police from car drivers suspected of impaired driving. The samples were never intended for pharmacokinetic studies. Nevertheless, we wanted to see if anything sensible could be said about the elimination rate at the time of blood sampling, in this specific population, by looking at only two samples taken a very short time apart. $\endgroup$
    – Peder Holman
    Commented Oct 16, 2021 at 14:24
  • $\begingroup$ I'm curious about the result of a regression (or fit incorporating $C_1$'s sampling uncertainty) $\log C_2 \sim \log C_1$; it it would be good to see that the data is consistent with the assumption that $C_2$ is a fixed proportion of $C_1$ (i.e. that the slope in this regression is consistent with 1; the intercept would correspond to the decayed proportion, assumed to be constant since $\Delta t$ is fixed). Since each concentration is non-negative, assuming log-normal sampling uncertainty seems reasonable. $\endgroup$
    – jwimberley
    Commented Oct 16, 2021 at 14:46
2
$\begingroup$

For question 2, there can be no exact answer with a log of normally distributed variables, since some of the variates will be negative.

However the ends of the distribution are managed, we can quickly approximate the result using the delta method with $f(X)=\ln(X)/0.5$, $\mu=1$, $\sigma=.09$: \begin{align} Var\Big[f(N(\mu,\sigma))\Big] &\simeq f'(\mu)^2\, \sigma^2\\ Var\Big[\ln(C_i)/0.5\Big]&\simeq 4(.09)^2\\ Var\!\left[\frac{\ln(C_1)-\ln(C_2)}{0.5}\right]&\simeq 8(.09)^2\\ SD\!\left[\frac{\ln(C_1)-\ln(C_2)}{0.5}\right]&\simeq 2\sqrt{2}(.09)=.255 \end{align}

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.