2
$\begingroup$

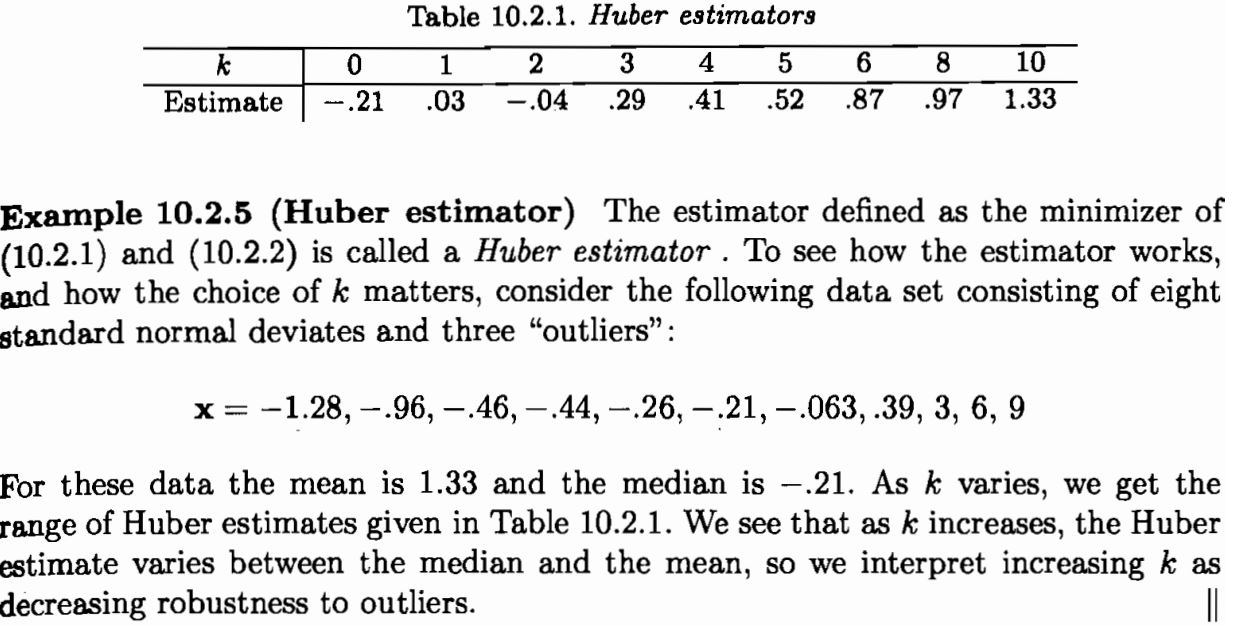
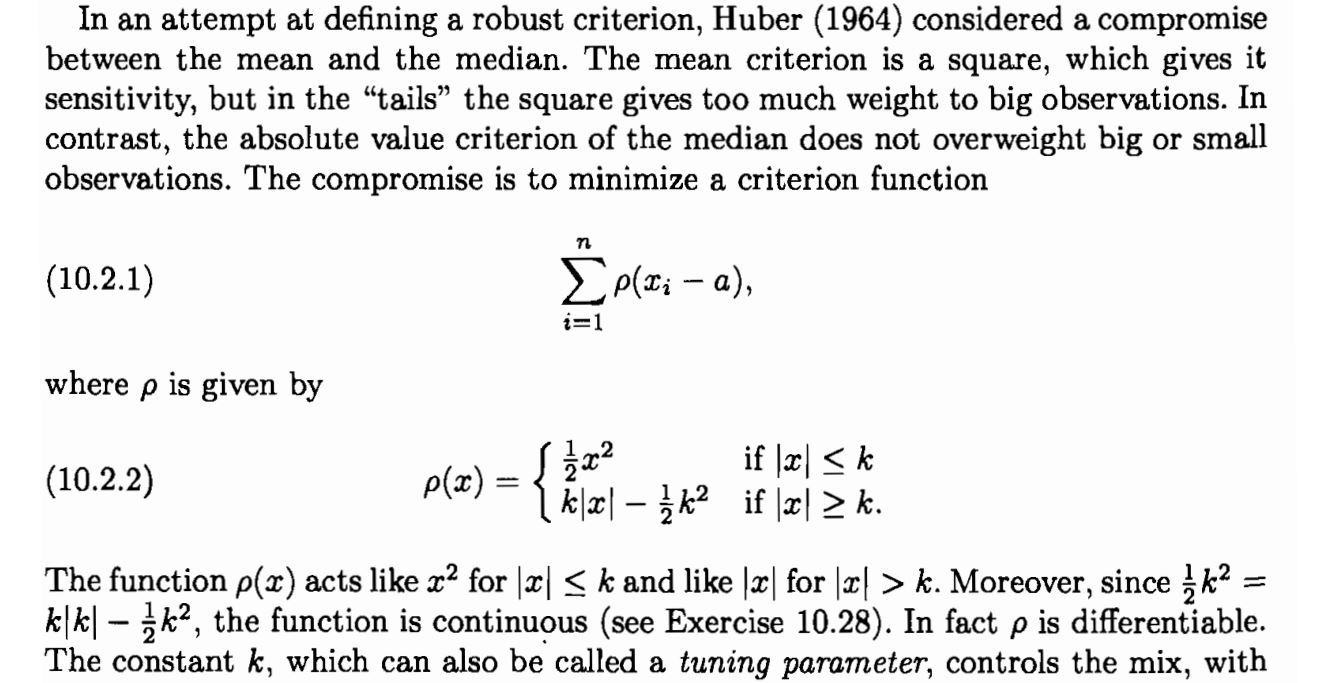
In Casella and Berger (page484), the following Huber loss is defined. enter image description here

Then on the next page, Table 10.2.1 shows the Huber estimator for different $k$:

enter image description here

In particular, $k=0$ gives the median, which doesn't make any sense. As when $k=0$, the Huber loss according to (10.2.2) is identically 0. But in order to get the median the huber loss has to be the absolute value (possibly with a shift). I guess the form of Huber loss that he gives here is wrong. What's the correct form of Huber loss then?

Thanks!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Equation 10.2.2 in Casella & Berger is correct. You are right of course that $\rho(x)$ is identically zero when $k=0$ but the estimate is nevertheless defined at $k=0$ in a limiting sense. The value of $a$ that minimizes the criterion function converges to the median as $k$ converges to zero from above. The estimate can be made arbitrarily close to the median by setting $k$ to a sufficiently small positive value.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks. This is very helpful! Do you have any good and detailed reference on the Huber loss or the Huber estimator? $\endgroup$
    – ExcitedSnail
    Commented Nov 8, 2021 at 9:40
  • 1
    $\begingroup$ @T34driver You don't consider Casella & Berger or Huber (1964) to be good references? One of the Huber's books would be my first thought. There are many Huber estimators. A Google Scholar search for "Huber loss" (with quotes) brings up over 6000 publications, but they are mostly written for a specialist mathematical research audience. $\endgroup$
    – Gordon Smyth
    Commented Nov 9, 2021 at 1:40
  • $\begingroup$ Thanks a lot, professor. Casella&Berger is the best book on statistical inference I have ever read of O(∩_∩)O, I was thinking about reference that is more specialized on Huber-type estimation. Will definitely check out Huber(1964). Many thanks again. $\endgroup$
    – ExcitedSnail
    Commented Nov 9, 2021 at 6:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.