Suppose that for $\theta ∈ (0, 1)$, $X$ is a continuous random variable with density $f_\theta(x) = \frac{3(1 − \theta)} {4\delta^3(\theta)} [\delta^2(\theta) − (x − \theta)^2]\mathbb{1}(|x − \theta| ≤ \delta(\theta)) + \frac{\theta}{2} \mathbb{1}(|x| ≤ 1)$ where $\delta(\theta) > 0$ for all $\theta$.
I'm struggling to show that the maximum likelihood estimator exists. Computing $f'$ and $f''$ gives nothing. Is there any hint? Thanks in advance.
The properties of the function $\delta(\theta)$ are important for the existence of a maximum likelihood estimate.
Example: let the observation be $x = 0$, then the likelihood function is
$$\mathcal{L}(\theta|x=0) = \theta \left(\frac{1}{2} \right)+ \left(1-\theta\right) \times \frac{3}{4\delta(\theta)} \left( 1 - \left(\frac{\theta}{\delta(\theta)}\right)^2\right)$$
Then for some suitably chosen $\delta(\theta)$ you can have a maximum in both $\theta = 1$, with value $0.5$, as well as in the point $\theta = 0$ with value $0.5$ as well if $\delta(0) = 3/2 $.
In the extreme case, possibly you could find a function $\delta(\theta)$ that makes the likelihood function constant in the entire range. That would require you to solve for a function $\delta(\theta)$ that makes the likelihood constant. Possibly one can solve the related differential equation, but the idea that the function can have maximum's in two points is already sufficient.
Your interval is not a closed interval. So you may have a situation where the likelihood function increases for $\theta \to 0$ or $\theta \to 1$, and a single point where the lowest upper bound is obtained does not exist.
Example: let the observation be $x = 0$, and $\delta(\theta) = 2\theta$ then
$$\mathcal{L}(\theta|x=0) = \theta \left(\frac{1}{2} \right)+ \left(1-\theta\right) \times \frac{9}{32\theta}$$
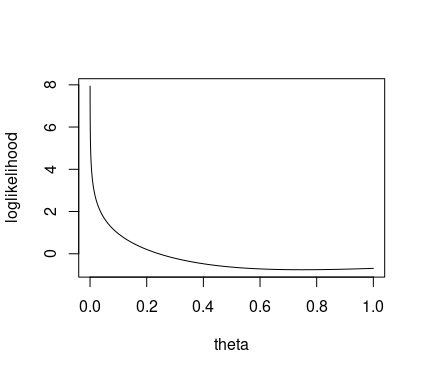
This situation, where the upper bound can be infinite can occur when $\delta(\theta)$ can approach $0$ (without actually being equal to $0$).
The situation, where the lowest upper bound is finite, but can still not be obtained can occur when the function is discontinuous or the interval is not closed. (the sufficient conditions of the extreme value theorem)
