3
$\begingroup$

Good day everyone, I was making an attempt on a self-study question on Confidence Interval:

The CEO of Micosaft Inc is considering the proposal of offering a child care program for its employees. As part of the feasibility study, the CEO wishes to estimate the mean weekly child-care cost of their employees. A sample of 60 employees who use child care reveals the following statistics:

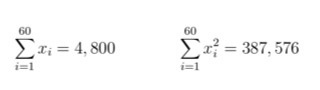
enter image description here

where xi is the weekly child-care cost of 1-th employees. Find a 95% confidence interval for the population mean.

My attempt as follows:

enter image description here

Unfortunately, my answer defers from the model answer of ***80 +_ 2.0111. Appreciate any guidance on where am I not doing right. I've looked at the question and my attempt multiple times without much success to find a way to correct it.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Although I cannot reproduce the "correct" answer, I can get close ($2.008$) by using a Student t distribution with $60-1$ degrees of freedom instead of a Normal distribution. Is the Student t also covered in this study material? $\endgroup$
    – whuber ♦
    Commented Apr 28, 2014 at 20:50
  • $\begingroup$ @whuber yes sir, it is. Studentized T Distribution is something I've read. $\endgroup$
    – user1275515
    Commented Apr 29, 2014 at 13:27

2 Answers 2

Reset to default
2
$\begingroup$

There's several minor errors here that will lead you into problems.

1) the sample standard deviation is not $\sigma$. By convention, Greek letters are for population parameters not sample quantities. You computed $s_{n-1}$, not $\sigma$. Call it $s$ and you won't be so likely to mislead yourself into:

2) You're using Z tables. You don't know $\sigma$, you estimated it, so you're dealing with a t-distribution as the basis for the interval*, not a normal distribution.

In this case (n=60) the distinction between the two isn't terribly large, but if you want to understand what you're doing, always treat $t$ as $t$ until the final step, even if $n$ is in the hundreds (that is, only bring the approximation of $t$ by a normal in at the very end, to help keep the concepts clearly distinct).

* specifically, the pivotal quantity from which the interval is derived has a t-distribution

Improve this answer
$\endgroup$
2
$\begingroup$

Update

Based on whuber's pointing out that you do not have the data, I get your result for the variance (it was in an unfamiliar form), but when we use the t distribution as whuber inquires after I get exactly the text's answer:

$s_{\bar{x}} = 1.00507$

$t_{\alpha/2,\text{ }\nu=59} = 2.00100$

95% CI: $80 \pm 2.01114$

Improve this answer
$\endgroup$
6
  • $\begingroup$ many thanks for your rapid reverts. I have attempted to use the above formulae, but I am still getting at the exact same output. I was wondering if there's anything fundamentally with how I did my standard deviation calculations? I've shared my working via picpaste.com/pics/image-12hEwqZw.1398713163.jpg $\endgroup$
    – user1275515
    Commented Apr 28, 2014 at 19:27
  • 1
    $\begingroup$ In addition, there's possibly a learning opportunity for you here: $(x_{i} - \bar{x})$ is the deviation of observation $x_{i}$ from the mean ($\bar{x}$). If you stare at the variance formula, you'll see that the variance is more or less the (artithmetic) mean of squared deviations (i.e. add up the number of squared deviations, and divide by the number of squared deviations, or, for the sample variance, divide by $n-1$, because of sampling uncertainty). $\endgroup$
    – Alexis
    Commented Apr 28, 2014 at 20:44
  • 1
    $\begingroup$ Alexis, I fear you may be leading the OP astray. Notice he has only the sum and sum of squares of the data; therefore an algebraically equivalent expression for the variance estimator is needed--and he is using a correct one and appears to be computing with it correctly. (Your first comment seems to overlook the fact that $\sum_i \bar{x} x_i = 0$ when computing the sum of squares of residuals.) $\endgroup$
    – whuber ♦
    Commented Apr 28, 2014 at 20:48
  • 1
    $\begingroup$ Hmmm you may be correct there, I missed that he didn't have the actual data. While $\sum{\varepsilon_{i}}=0$ I don't think $\sum{\bar{x}x} = 0$, for example: $x \in \{1,2,3\}$, $\bar{x} = 2$, $\bar{x}x \in \{2,4,6\}$, and $\sum{\bar{x}x}=12 \ne 0$ $\endgroup$
    – Alexis
    Commented Apr 28, 2014 at 21:04
  • 1
    $\begingroup$ You're right: I meant to write that $\sum\bar{x}(x_i-\bar{x})=0$. With that in mind you need to replace the "$\ne$" in your first comment with an "$=$" (assuming that by "$x$" you meant "$\bar x$"). Nevertheless, thank you for checking the calculation and big +1 for editing your answer to help the O.P.! $\endgroup$
    – whuber ♦
    Commented Apr 28, 2014 at 22:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.