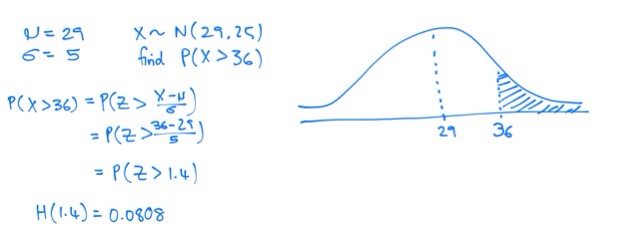2
$\begingroup$

Good day everyone, I am currently working on a self-study question:

Question

The number of viewers of a television show has a mean of 29 million with a standard deviation of 5 million. Assume this distribution follows a normal distribution. What is the probability that at least one of the four shows has more than 36 million viewers in the next four shows. Assume the number of viewers in each show are independent.

My Attempt

enter image description here

However, it seemed that the answer is not correct from that of the answer key (0.2861). Appreciate some guidance on this. I know I'm missing out on something, but I just can't figure out what.

Improve this question
$\endgroup$
3
  • $\begingroup$ You don't seem to be dealing with the "at least one of the four" part. You deal with that part using basic probability calculations. $\endgroup$
    – Glen_b
    Commented Apr 27, 2014 at 16:15
  • $\begingroup$ Thanks for the pointer Glen. I attempted to take (0.0808)^4 but to no avail either. Guess it is not as simple as this. I was wondering if Binomial is something that can be used here? With success means having more than 36 million viewers, and failure otherwise. Many thanks once again. $\endgroup$
    – user1275515
    Commented Apr 27, 2014 at 16:19
  • $\begingroup$ Looks like I've managed to get the answer using Binomial. Many thanks for the advice Glen. I will post my solution shortly $\endgroup$
    – user1275515
    Commented Apr 27, 2014 at 16:22

1 Answer 1

Reset to default
1
$\begingroup$

enter image description here

After taking in Glen's advice that I am missing the "at least one of four" part on the question, I managed to use the Binomial function to solve the equation.

What I did was to count an instance of having more than 36 million viewers as a success, and failure otherwise.

Using the Binomial function: $P(X=k) = C_n^k \cdot p^k \cdot (1 - p)^{n-k}$

To find $P(Y>0)$, we can compute $1 - P(Y=0)$.

Therefore, \begin{align*} P(Y > 0) &= 1 - P(Y=0) \\ &= 1 - C_4^0 \cdot 0.0808^0 \cdot (1 - 0.0808)^4 \\ &= 0.286096. \end{align*}

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.