1
$\begingroup$

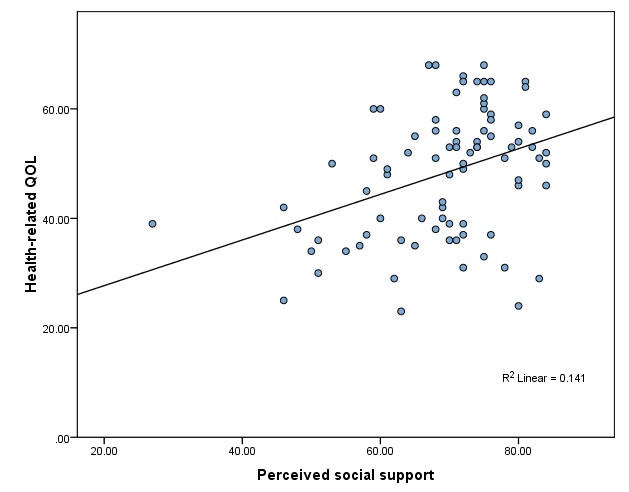
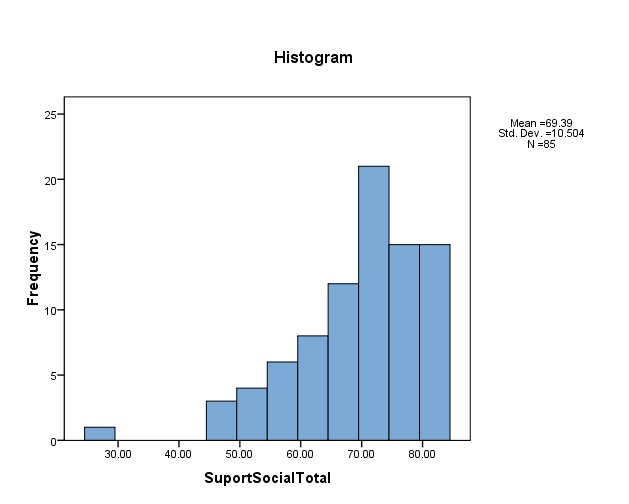
I am confused. My scatterplot says that the relationship between the two variables is not linear, so I can't use Pearson's correlation and neither is it monotonic, so I can't use Spearman's correlation. From what I've learned these are assumptions that you have to meet so you can run those statistical tests. And considering that I don't meet any, I don't know what to do. Also, my variables are continuous.

Thank you!

enter image description here

enter image description here

Improve this question
$\endgroup$
11
  • 5
    $\begingroup$ Can you say more about your situation, your data, & your goals? Can you paste your scatterplot into your question? $\endgroup$
    – gung - Reinstate Monica
    Commented Jun 14, 2015 at 17:28
  • $\begingroup$ What are you trying to find out/show? $\endgroup$
    – Glen_b
    Commented Jun 15, 2015 at 3:04
  • 2
    $\begingroup$ Neither Pearson nor Spearman correlation is absurd or inapplicable here. What's important is their value, in each case small positive (presumably), which tells you that you have a weak relationship here. Whether there is underneath that something of clinical importance which is obscured by the effects of other variables is not something we can tell. I wouldn't call the skewness "terrible"; it's just negative skewness that makes sense. $\endgroup$
    – Nick Cox
    Commented Jun 15, 2015 at 18:21
  • 1
    $\begingroup$ If linearity were a prerequisite for running Pearson's correlation, then only perfect correlations would be calculated, and we would learn nothing from them. On the contrary, the main role of Pearson correlation is to measure how well the data could be summarized by a linear relationship. Similar remarks apply to Spearman and monotonicity. $\endgroup$
    – Nick Cox
    Commented Jun 15, 2015 at 18:23
  • 1
    $\begingroup$ You already used it, tacitly. Your $R^2$ is 0.141, so it's about 0.375 or 0.376. $\endgroup$
    – Nick Cox
    Commented Jun 15, 2015 at 19:29

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.