Two Way Anova with Mixed Effects Model

Question

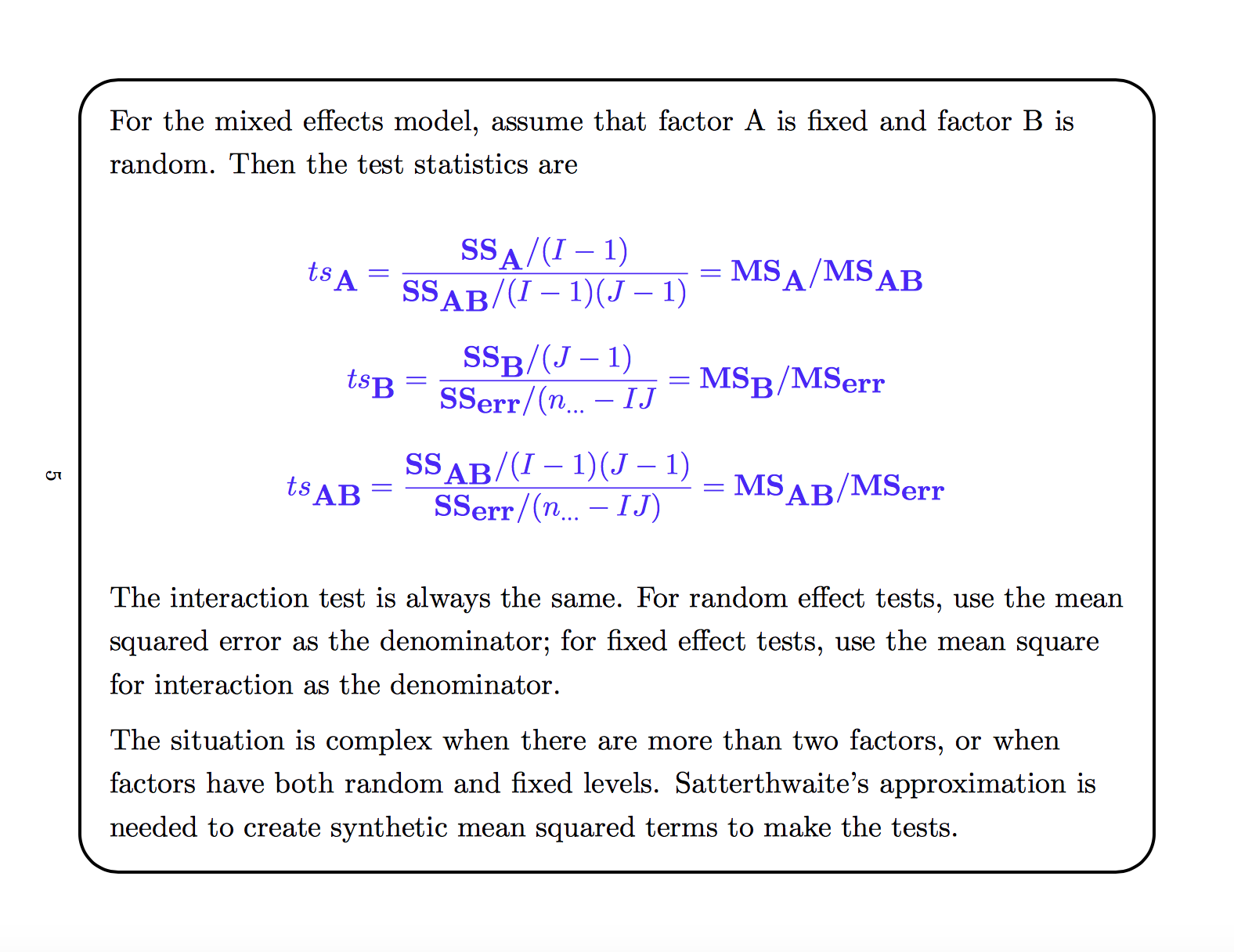
I have been trying to understand the differences and relationship between fixed, random, and mixed effect models. I have found the following slides (link: http://www2.stat.duke.edu/~banks/111-lectures.dir/lect21a.pdf).

So, when we assume a factor has fixed effect, then the denominator of the test statistic is $MS_{err}$, and if we assume the factor is random, the denominator is $MS_{AB}$. However, they change the role in the mixed effects model (in the second figure). Un the mixed effect model A is a fixed factor and B is a random factor. However, the denominator of the corresponding test statistics are $MS_{AB}$ and $MS_{err}$, respectively.

Can someone explain the rationale behind this, please?

Answer 1

Here is a conceptual description. I'll leave the math to others.

If B were fixed then you would assess the effect of A for the specific values of B in the experiment. Since B is random, you are interested in generalizing the effect of A to all levels of B. The more the effect of A differs as a function of the level of B, the harder it is to generalize. That's why the AxB interaction is in the denominator.