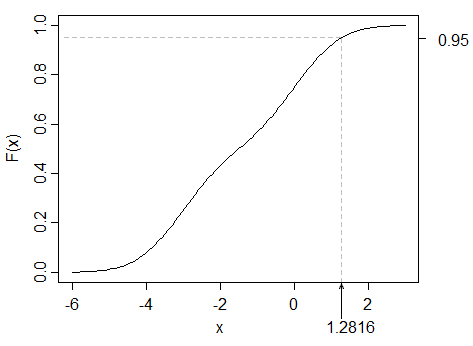
On R, I have created a mixture distribution via a convex combination of a standard normal distribution, and a normal distribution with mean -3, variance 1 (i.e. subtracting 3 from a standard normal distribution).
In doing so, I have observed that the difference between absolute values of the upper quantiles (e.g. 95%) of the mixture distribution and a standard normal distribution are smaller than the same difference at lower quantiles (e.g. 5%).
I have an application for this result in economics, but other than looking at the plots of the distributions and using intuition, I have no way of explaning why this is the case. Is anyone able to offer an explanation as to why I observe this result mathematically?
Also, is this a result that holds generally when you form a mixture distribution via a convex combination of a standard normal distribution, and a normal distribution with lower mean?