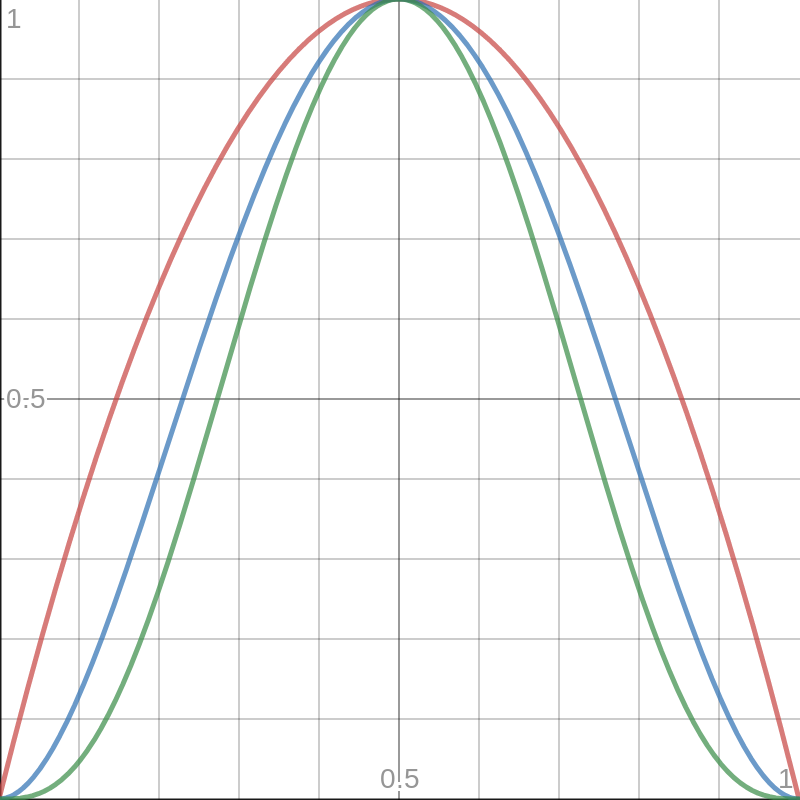
I came-up with a type of central tendency which is a weighted mean. The weighting is based on percentile, with values closer to the median having a higher weight. It's similar to the idea of a truncated mean, but it's a soft approach to dealing with outliers. For example, if x is the percentile of each value in the set, the weights can be determined with these 3 functions
x(x-1)
(x(x-1))²
(x(x-1))³
I'm sure I'm not the first to come-up with this. Is there an existing term for this kind of central tendency?