I would appreciate some help in deciding the analysis method for my research.
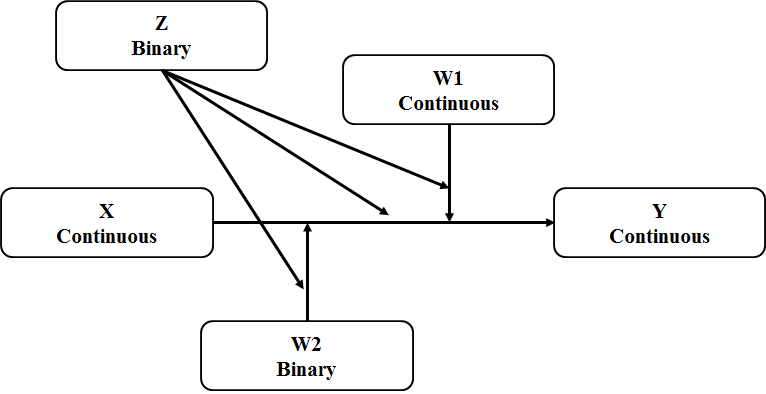
I have 7 variables: one DV, one IV, two moderators, one moderator/moderating moderator plus 2 controls. My overall sample size is 100 which is divided by the levels of Z (two countries) into 46 and 54. The DV, IV and one of the moderators are Likert-scale data (7 points), the other moderator and moderator/moderating moderator are binary.
My main purpose is to investigate the differences between the two countries regarding the relationships among the other variables.
I tried two analysis methods which I find okay but not sure which one is the best. The first one is regression analysis using R and the second is PLS-SEM using SmartPLS.
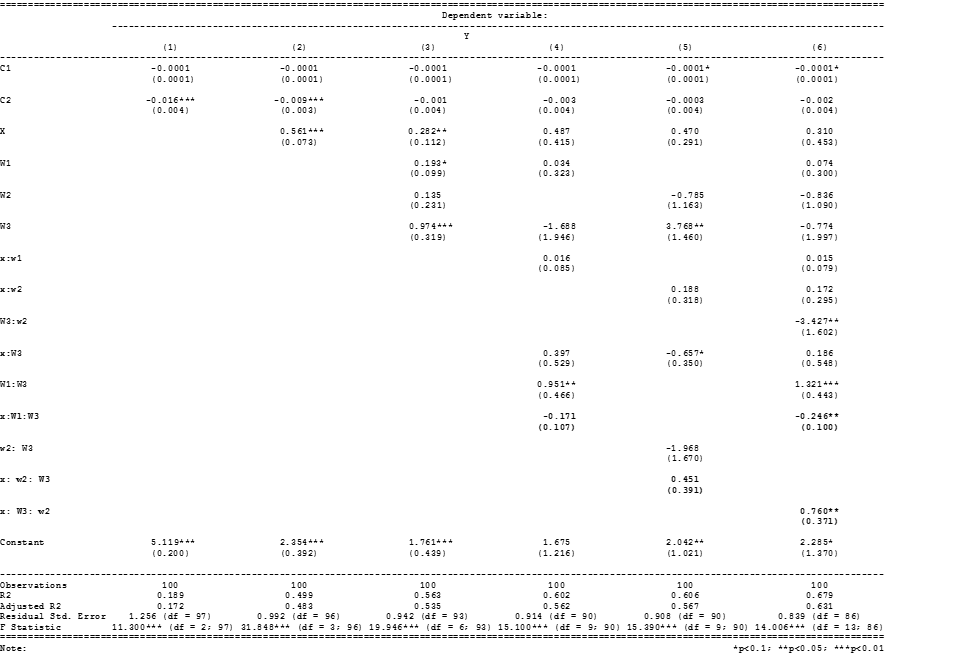
For the regression analysis, I ran the following models (ignore model 2): Note: W3 = Z m1 <- lm (Y ~ C1 + C2, data = DataR100C) m3 <- lm (Y ~ C1 + C2 + X + W1 + W2 + Z, data = DataR100C) m4 <- lm (Y ~ C1 + C2 + X*W1*Z, data = DataR100C) m5 <- lm (Y ~ C1 + C2 + X*W2*Z, data = DataR100C) m6 <- lm (Y ~ C1 + C2 + X*W1*Z + X*W2*Z, data = DataR100C)
The results are as follows:
First, I would like some help with the interpretation. I see that both 3-way interactions are significant when combined but not separately. what does this mean?!
For the second method; PLS-SEM, I added the two interaction terms to the model then ran bootstrapping analysis, then I ran MGA between the two countries (Z) to detect multigroup differences.
Which method is better to best present my main focus, having in mind the small sample size? are there other methods I should try?
Thanks.