0
$\begingroup$

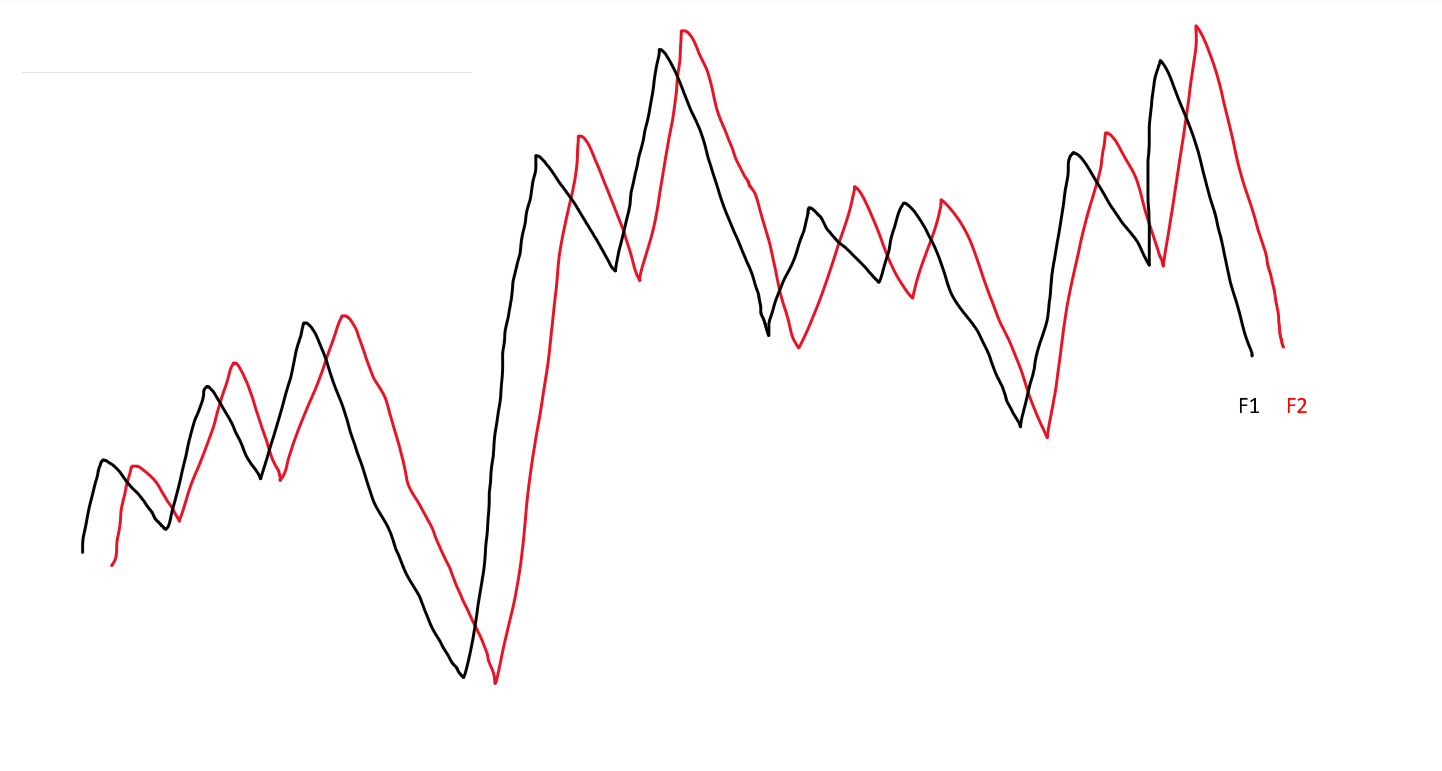
I am wondering if these two lines, F1 and F2, representing time series, would still be considered "random walk", once the relationship between the two was discovered? Could this relationship ever even happen if a process was truly random walk? What would these two time series be considered instead, if not random walk? Is there a way to test if a trend is a random walk or has a deterministic trend? In the examples I have seen this seems to be done manually.

enter image description here

Please excuse the quick drawings, I am trying to learn about stationary vs. non-stationary data and I'm trying to check my understanding of what a "random walk" is. My maths knowledge is not advanced so please keep any explanation simple and understandable - thank you!

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

"Random" and "uncorrelated", or "independent", are not the same thing. Say that you throw a coin and record heads as 1 and tails as 0 in column A of your Excel spreadsheet, while in column B you record tails as 1 and heads as 0. Both series would be equally random (tossing heads is equally random event like tossing tails), but would be perfectly correlated. You seem to be referring to popular, rather then formal definition of "randomness".

Improve this answer
$\endgroup$
4
  • $\begingroup$ Following the formal definition of random walk, should I infer from your answer that if there is a causal relationship between F1 and F2 they are not a random walk? $\endgroup$
    – Darcey BM
    Commented Apr 30, 2020 at 15:16
  • $\begingroup$ @DarceyBM what definition exactly? You don’t give us much details so it is hard to say anything more. $\endgroup$
    – Tim
    Commented Apr 30, 2020 at 17:29
  • $\begingroup$ Definition of what? I am trying to understand the fundamental relationships of these things, I have said that I am very new to this. I am not deliberately excluding details, I am simply unclear on what details are needed for someone to be able to help! $\endgroup$
    – Darcey BM
    Commented May 1, 2020 at 13:12
  • $\begingroup$ You said “formal definition of random walk”, my question is what definition you have in mind? $\endgroup$
    – Tim
    Commented May 1, 2020 at 16:29
0
$\begingroup$

Based on your example, it seems like there is a correlation between F1 and F2-- note that F2 seems to follow the movement of F1.

I cannot confirm if practically we won't have this situation.

Talking about Random walk theory, by definition, is any time series that follows random walk properties, that is, it is not stationary; its variance increases over time and covariance changes along the time.

If your F1 has these properties then it is considered to be a random walk. Visually, you also can check this with acf function in R.

Thanks Tim for pointing out that I should describe the relationship as correlation, and not causality

Improve this answer
$\endgroup$
3
  • $\begingroup$ Correlation is not causation. $\endgroup$
    – Tim
    Commented Apr 29, 2020 at 22:26
  • $\begingroup$ Thanks for pointing this out @Tim $\endgroup$
    – Apichart Thanomkiet
    Commented Apr 29, 2020 at 22:30
  • $\begingroup$ Time series that satisfy the statistical criteria for a random walk can be related. $\endgroup$
    – zbicyclist
    Commented Apr 30, 2020 at 14:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.