3
$\begingroup$

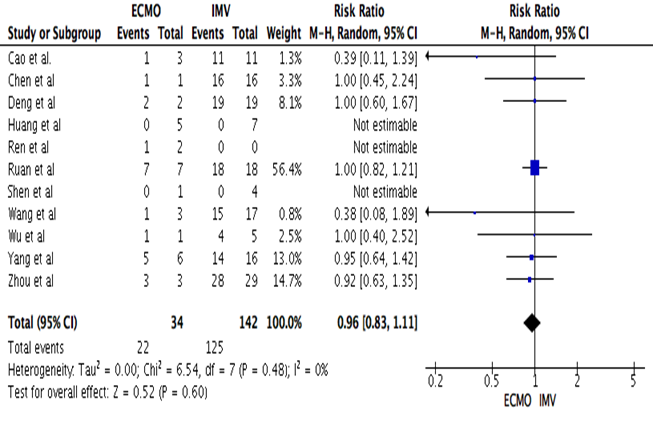
I am doing a meta-analysis and struggling with the relative risk values. Looking at the forest plot and the RR for some relative risks I can tell it is incorrect. i.e first study rr should be 0.33. I have entered numbers from other metaanalysis and it seems to generate the right RR, so how come it doesn't seem to be working with my studies. Some of the RR is right but some off.

enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

As clearly stated in the Cochrane Handbook (https://handbook-5-1.cochrane.org/chapter_9/9_4_4_1_mantel_haenszel_methods.htm):

The Mantel-Haenszel methods (Mantel 1959, Greenland 1985) are the default fixed-effect methods of meta-analysis programmed in RevMan. When data are sparse, either in terms of event rates being low or study size being small, the estimates of the standard errors of the effect estimates that are used in the inverse variance methods may be poor. Mantel-Haenszel methods use a different weighting scheme that depends upon which effect measure (e.g. risk ratio, odds ratio, risk difference) is being used. They have been shown to have better statistical properties when there are few events. As this is a common situation in Cochrane reviews, the Mantel-Haenszel method is generally preferable to the inverse variance method. In other situations the two methods give similar estimates.

I recommend you to switch to another method, and you can also switch to Peto's odds ratio for such sparse events. Check here for additional info from Cochrane https://handbook-5-1.cochrane.org/chapter_9/9_4_4_meta_analysis_of_dichotomous_outcomes.htm, and here for the very comprehensive meta R package https://cran.r-project.org/web/packages/meta/meta.pdf.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Thank you for your prompt reply Joe. Im a newbie to stats so bear with. Cochrane handbook says Peto OR 'when intervention effects are small (odds ratios are close to one), events are not particularly common and the studies have similar numbers in experimental and control groups.'. Most of my studies the control group is considerably larger than the exposure group. Is there a resource, other than that handbook, which gives a comprehensive (but simple) overview of commonly used methods for meta-analysis. $\endgroup$
    – Bilal Ali
    Commented May 21, 2020 at 23:03
3
$\begingroup$

The reason is that a 'continuity correction' is applied in those studies where you are seeing a discrepancy. This correction involves adding 0.5 to the number of events and 1 to the total. For example, in the first study:

> round((1.5 / 4) / (11.5 / 12), 2)
[1] 0.39

You actually have a very common (not sparse) outcome (i.e., often the number of events is equal to the total). Still, as suggested by @Joe_74, I would consider switching to a different method for analyzing these data (e.g., the Mantel-Haenszel method or using a logistic mixed-effects model).

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Thank you for your reply. I think I may need to change my method as you state. This is MH rando effects (which I thought was most reliable for starting the Meta-analysis). Dont think logistic mixed effects is an option on Revman .Available options are peto, MH, and inverse variance. Is there an overview when I use which one $\endgroup$
    – Bilal Ali
    Commented May 21, 2020 at 23:07
  • 1
    $\begingroup$ Just use a MH fixed-effects model. That has the desirable properties of the MH method (as opposed to the 'MH random-effects model' that is available in Review Manager, which is a Cochrane invention and lacks proper motivation). $\endgroup$
    – Wolfgang
    Commented May 22, 2020 at 7:58
  • 1
    $\begingroup$ Dear Bilal, if you are a newbie I am just a MA lover... Do as Wolfgang says, he is the real expert $\endgroup$
    – Giuseppe Biondi-Zoccai
    Commented May 22, 2020 at 8:43

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.