How rigorous do you want the exposition to be? In simple terms, even though we often talk about "dependence" and "correlation" in intuitive terms, they have formal definitions. In particular, Kendall's tau has a formal mathematical definition, and it measures the probability of concordance minus the probability of disconcordance, where concordance between $(X,Y)$ is defined as the probability that $X_1 > X_2$ and $Y_1 > Y_2$ jointly, where $(X_1,Y_1)$, $(X_2,Y_2)$ are independent random variables with the same distribution of $(X,Y)$. As such, even if $(X,Y)$ have a high value for kendall's tau, they can certainly have little concordance at the tails (or any other part of the distribution) as long as the mass of being at those parts are low enough. If I have no association at a certain part of the distribution, but the density is really low, then an overall measure of kendall's tau may say little about the dependence at certain areas.
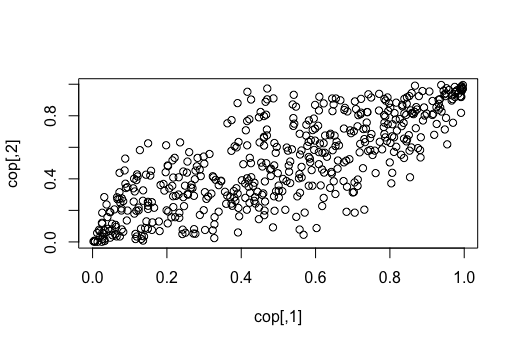
To answer your question more explicitly, if you know that the copula is a Gaussian copula, then knowing the parameter $.8$ is in some sense all you need to know. A commonly studied feature of copulas is the upper (lower) tail dependence, which precisely measures the 'relationship' of the copula at the tails. It can be shown that for gaussian copulas, the tail dependence is $0$. So in some sense, this is why the correlation in the middle does not apply at the extremes--it has to do with your choice of copula. For a rigorous proof of this, and also an intuitive visualization, check out this post:
Why is Gaussian Copula's Tail Dependence Zero?
Generally, copulas measure arbitrarily complicated and intricate relationships, and there's no reason why correlation in the middle implies anything about correlation at the tails, and measures such as Kendall's tau provide information about the overall 'relationship.' Maybe not the best comparison, but it's sort of similar to how for a random variable, the mean and variance provide information about the variable, but it says little about tail behavior, and so just how tail dependence exits as a concept for copulas, so does concepts like kurtosis for a random variable.